Page 66 - Start Up Mathematics_6

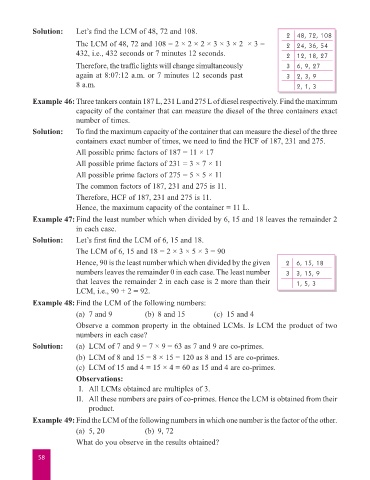
Solution: Let’s find the LCM of 48, 72 and 108.
2 48, 72, 108
The LCM of 48, 72 and 108 = 2 × 2 × 2 × 3 × 3 × 2 × 3 = 2 24, 36, 54
432, i.e., 432 seconds or 7 minutes 12 seconds. 2 12, 18, 27
Therefore, the traffic lights will change simultaneously 3 6, 9, 27
again at 8:07:12 a.m. or 7 minutes 12 seconds past 3 2, 3, 9
8 a.m. 2, 1, 3

Example 46: Three tankers contain 187 L, 231 L and 275 L of diesel respectively. Find the maximum
capacity of the container that can measure the diesel of the three containers exact
number of times.
Solution: To find the maximum capacity of the container that can measure the diesel of the three
containers exact number of times, we need to find the HCF of 187, 231 and 275.
All possible prime factors of 187 = 11 × 17

All possible prime factors of 231 = 3 × 7 × 11
All possible prime factors of 275 = 5 × 5 × 11
The common factors of 187, 231 and 275 is 11.

Therefore, HCF of 187, 231 and 275 is 11.
Hence, the maximum capacity of the container = 11 L.
Example 47: Find the least number which when divided by 6, 15 and 18 leaves the remainder 2
in each case.
Solution: Let’s first find the LCM of 6, 15 and 18.

The LCM of 6, 15 and 18 = 2 × 3 × 5 × 3 = 90
Hence, 90 is the least number which when divided by the given 2 6, 15, 18
numbers leaves the remainder 0 in each case. The least number 3 3, 15, 9
that leaves the remainder 2 in each case is 2 more than their 1, 5, 3
LCM, i.e., 90 + 2 = 92.
Example 48: Find the LCM of the following numbers:

(a) 7 and 9 (b) 8 and 15 (c) 15 and 4
Observe a common property in the obtained LCMs. Is LCM the product of two
numbers in each case?
Solution: (a) LCM of 7 and 9 = 7 × 9 = 63 as 7 and 9 are co-primes.

(b) LCM of 8 and 15 = 8 × 15 = 120 as 8 and 15 are co-primes.
(c) LCM of 15 and 4 = 15 × 4 = 60 as 15 and 4 are co-primes.
Observations:
I. All LCMs obtained are multiples of 3.
II. All these numbers are pairs of co-primes. Hence the LCM is obtained from their
product.

Example 49: Find the LCM of the following numbers in which one number is the factor of the other.
(a) 5, 20 (b) 9, 72
What do you observe in the results obtained?

58