Page 63 - Start Up Mathematics_6

Example 34: Find the HCF of 70, 105 and 75 by listing factors.

Solution: All possible factors of 70 are 1 , 2, 5 , 7, 10, 14, 35 and 70.
All possible factors of 105 are 1 , 3, 5 , 7, 15, 21, 35 and 105.

All possible factors of 75 are 1 , 3, 5 , 15, 25 and 75.
The common factors of the given numbers are 1 and 5.
The HCF of the numbers is the highest of their common factors, i.e., 5.

HCF by prime factorization
Step 1: Find prime factors of each of the given numbers.

Step 2: Identify the common prime factors of the given number.
Step 3: Multiply the common prime factors to obtain the HCF.
Example 35: Find the HCF of the following numbers by prime factorization method:
(a) 18, 60 (b) 91, 112, 49
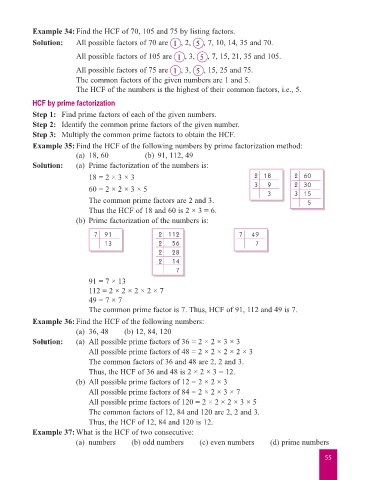
Solution: (a) Prime factorization of the numbers is:

18 = 2 × 3 × 3 2 18 2 60
60 = 2 × 2 × 3 × 5 3 9 2 30
3 15
3

The common prime factors are 2 and 3. 5
Thus the HCF of 18 and 60 is 2 × 3 = 6.
(b) Prime factorization of the numbers is:

7 91 2 112 7 49
13 2 56 7
2 28
2 14
7
91 = 7 × 13
112 = 2 × 2 × 2 × 2 × 7
49 = 7 × 7
The common prime factor is 7. Thus, HCF of 91, 112 and 49 is 7.
Example 36: Find the HCF of the following numbers:
(a) 36, 48 (b) 12, 84, 120
Solution: (a) All possible prime factors of 36 = 2 × 2 × 3 × 3
All possible prime factors of 48 = 2 × 2 × 2 × 2 × 3

The common factors of 36 and 48 are 2, 2 and 3.
Thus, the HCF of 36 and 48 is 2 × 2 × 3 = 12.
(b) All possible prime factors of 12 = 2 × 2 × 3
All possible prime factors of 84 = 2 × 2 × 3 × 7
All possible prime factors of 120 = 2 × 2 × 2 × 3 × 5
The common factors of 12, 84 and 120 are 2, 2 and 3.
Thus, the HCF of 12, 84 and 120 is 12.
Example 37: What is the HCF of two consecutive:
(a) numbers (b) odd numbers (c) even numbers (d) prime numbers

55