Page 292 - Start Up Mathematics_6

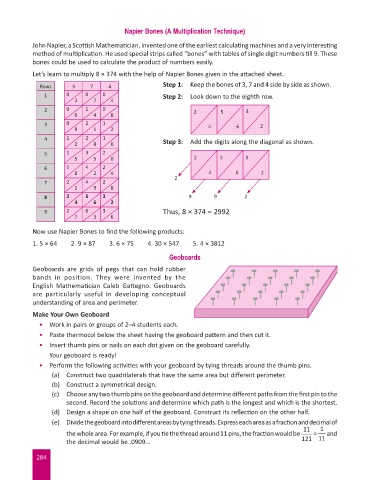
Napier Bones (A Multiplication Technique)
John Napier, a Scottish Mathematician, invented one of the earliest calculating machines and a very interesting
method of multiplication. He used special strips called “bones” with tables of single digit numbers till 9. These
bones could be used to calculate the product of numbers easily.
Let’s learn to multiply 8 × 374 with the help of Napier Bones given in the attached sheet.

Rows 3 7 4 Step 1: Keep the bones of 3, 7 and 4 side by side as shown.
1 0 0 0 Step 2: Look down to the eighth row.
3 7 4
2 0 1 0 2 5 3
6 4 8
3 0 2 1 4 6 2
9 1 2
4 1 2 1 Step 3: Add the digits along the diagonal as shown.
2 8 6
5 1 3 2
5 5 0 2 5 3
6 1 4 2
8 2 4 4 6 2
7 2 4 2 2
1 9 8
8 2 5 3 9 9 2
4 6 2
9 2 6 3 Thus, 8 × 374 = 2992
7 3 6

Now use Napier Bones to find the following products:
1. 5 × 64 2. 9 × 87 3. 6 × 75 4. 30 × 547 5. 4 × 3812

Geoboards
Geoboards are grids of pegs that can hold rubber
bands in position. They were invented by the
English Mathematician Caleb Gattegno. Geoboards
are particularly useful in developing conceptual
understanding of area and perimeter.
Make Your Own Geoboard
• Work in pairs or groups of 2–4 students each.
• Paste thermocol below the sheet having the geoboard pattern and then cut it.
• Insert thumb pins or nails on each dot given on the geoboard carefully.
Your geoboard is ready!
• Perform the following activities with your geoboard by tying threads around the thumb pins.
(a) Construct two quadrilaterals that have the same area but different perimeter.
(b) Construct a symmetrical design.
(c) Choose any two thumb pins on the geoboard and determine different paths from the first pin to the
second. Record the solutions and determine which path is the longest and which is the shortest.
(d) Design a shape on one half of the geoboard. Construct its reflection on the other half.
(e) Divide the geoboard into different areas by tying threads. Express each area as a fraction and decimal of
11 1
the whole area. For example, if you tie the thread around 11 pins, the fraction would be = and
the decimal would be .0909... 121 11

284