Page 141 - Start Up Mathematics_6

1 2 3 4 5
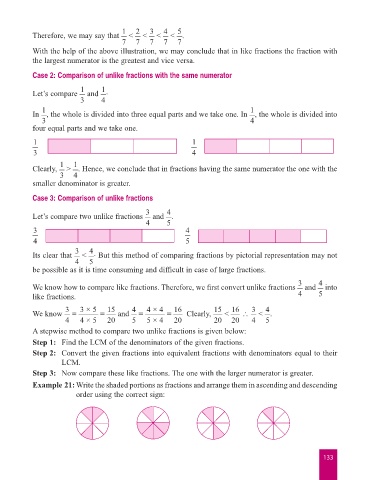
Therefore, we may say that < < < < .
7 7 7 7 7
With the help of the above illustration, we may conclude that in like fractions the fraction with
the largest numerator is the greatest and vice versa.

Case 2: Comparison of unlike fractions with the same numerator

1
Let’s compare and 1 .
3 4
1
1
In , the whole is divided into three equal parts and we take one. In , the whole is divided into
3 4
four equal parts and we take one.
1
1
3 4

1
1
Clearly, > . Hence, we conclude that in fractions having the same numerator the one with the
3 4
smaller denominator is greater.

Case 3: Comparison of unlike fractions

3 4
Let’s compare two unlike fractions and .
4 5
3 4

4 5
3 4
.
Its clear that < But this method of comparing fractions by pictorial representation may not
4 5
be possible as it is time consuming and difficult in case of large fractions.
3 4
We know how to compare like fractions. Therefore, we first convert unlike fractions and into
like fractions. 4 5

3 3 × 5 15 4 4 × 4 16 15 16 3 4
We know = = and = = Clearly, < ∴ < .
4 4 × 5 20 5 5 × 4 20 20 20 4 5
A stepwise method to compare two unlike fractions is given below:

Step 1: Find the LCM of the denominators of the given fractions.
Step 2: Convert the given fractions into equivalent fractions with denominators equal to their
LCM.
Step 3: Now compare these like fractions. The one with the larger numerator is greater.
Example 21: Write the shaded portions as fractions and arrange them in ascending and descending
order using the correct sign:

133