Page 123 - Start Up Mathematics_6

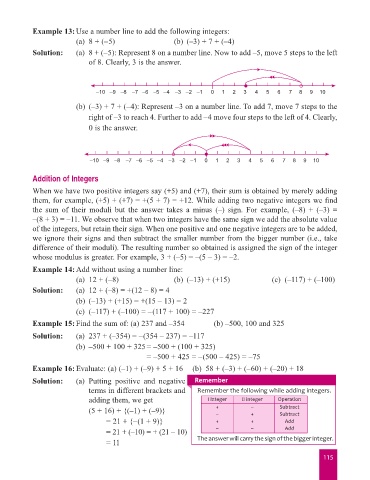
Example 13: Use a number line to add the following integers:
(a) 8 + (–5) (b) (–3) + 7 + (–4)

Solution: (a) 8 + (–5): Represent 8 on a number line. Now to add –5, move 5 steps to the left
of 8. Clearly, 3 is the answer.

–10 –9 –8 –7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7 8 9 10

(b) (–3) + 7 + (–4): Represent –3 on a number line. To add 7, move 7 steps to the
right of –3 to reach 4. Further to add –4 move four steps to the left of 4. Clearly,
0 is the answer.

–10 –9 –8 –7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7 8 9 10

Addition of Integers
When we have two positive integers say (+5) and (+7), their sum is obtained by merely adding
them, for example, (+5) + (+7) = +(5 + 7) = +12. While adding two negative integers we find
the sum of their moduli but the answer takes a minus (–) sign. For example, (–8) + (–3) =
–(8 + 3) = –11. We observe that when two integers have the same sign we add the absolute value
of the integers, but retain their sign. When one positive and one negative integers are to be added,
we ignore their signs and then subtract the smaller number from the bigger number (i.e., take
difference of their moduli). The resulting number so obtained is assigned the sign of the integer
whose modulus is greater. For example, 3 + (–5) = –(5 – 3) = –2.
Example 14: Add without using a number line:
(a) 12 + (–8) (b) (–13) + (+15) (c) (–117) + (–100)

Solution: (a) 12 + (–8) = +(12 – 8) = 4
(b) (–13) + (+15) = +(15 – 13) = 2
(c) (–117) + (–100) = –(117 + 100) = –227

Example 15: Find the sum of: (a) 237 and –354 (b) –500, 100 and 325
Solution: (a) 237 + (–354) = –(354 – 237) = –117
(b) –500 + 100 + 325 = –500 + (100 + 325)
= –500 + 425 = –(500 – 425) = –75

Example 16: Evaluate: (a) (–1) + (–9) + 5 + 16 (b) 58 + (–3) + ( –60) + (–20) + 18

Solution: (a) Putting positive and negative Remember
terms in different brackets and Remember the following while adding integers.
adding them, we get I integer II integer Operation

(5 + 16) + {(–1) + (–9)} + – Subtract
–
+
Subtract
= 21 + {–(1 + 9)} + + Add
= 21 + (–10) = + (21 – 10) – – Add
= 11 The answer will carry the sign of the bigger integer.

115