Page 107 - Start Up Mathematics_5

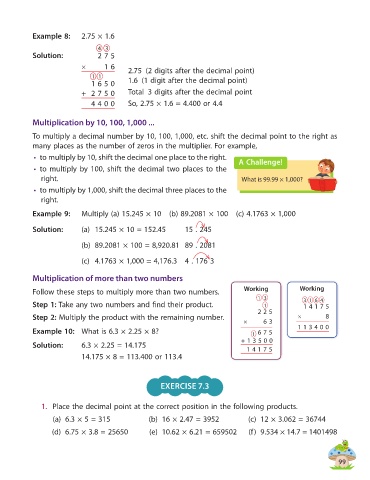
Example 8: 2.75 × 1.6

4 3
Solution: 2 7 5

× 1 6 2.75 (2 digits after the decimal point)
1
1
1 6 5 0 1.6 (1 digit after the decimal point)
+ 2 7 5 0 Total 3 digits after the decimal point
4 4 0 0 So, 2.75 × 1.6 = 4.400 or 4.4

Multiplication by 10, 100, 1,000 ...

To multiply a decimal number by 10, 100, 1,000, etc. shift the decimal point to the right as
many places as the number of zeros in the multiplier. For example,
• to multiply by 10, shift the decimal one place to the right. A Challenge!

• to multiply by 100, shift the decimal two places to the
right. What is 99.99 × 1,000?
• to multiply by 1,000, shift the decimal three places to the
right.

Example 9: Multiply (a) 15.245 × 10 (b) 89.2081 × 100 (c) 4.1763 × 1,000

Solution: (a) 15.245 × 10 = 152.45 15 . 245

(b) 89.2081 × 100 = 8,920.81 89 . 2081

(c) 4.1763 × 1,000 = 4,176.3 4 . 176 3

Multiplication of more than two numbers

Follow these steps to multiply more than two numbers. Working Working
1 3 3 1 6 4
Step 1: Take any two numbers and find their product. 1 1 4 1 7 5
2 2 5
Step 2: Multiply the product with the remaining number. × 8
× 6 3
Example 10: What is 6.3 × 2.25 × 8? 1 6 7 5 1 1 3 4 0 0
+ 1 3 5 0 0
Solution: 6.3 × 2.25 = 14.175 1 4 1 7 5
14.175 × 8 = 113.400 or 113.4

EXERCISE 7.3

1. Place the decimal point at the correct position in the following products.
(a) 6.3 × 5 = 315 (b) 16 × 2.47 = 3952 (c) 12 × 3.062 = 36744

(d) 6.75 × 3.8 = 25650 (e) 10.62 × 6.21 = 659502 (f) 9.534 × 14.7 = 1401498

99