Page 70 - ICSE Math 8

Example 7: Solve MN + NM = PMP, where MN and NM are 2-digit numbers and PMP is a 3-digit number.
Solution: Here MN is a 2-digit number and NM is obtained by reversing its digits.
Now, MN + NM = PMP
fi (10M + N) + (10N + M) = PMP
fi 11M + 11N = PMP fi 11(M + N) = PMP
Now, M + N cannot exceed 18 (as per the definition of generalized form of a number, M and
N can maximum be 9 each).
So, PMP cannot exceed 11 × 18 = 198 and should be a multiple of 11.
Let’s take all the 3-digit numbers less than 198 which are multiple of 11.
110, 121, 132, 143, 154, 165, 176, 187, 198
Among these numbers only 121 is a 3-digit number whose ones and hundreds digits are
same.
\ P = 1 and M = 2
121
Now, 11(2 + N) = 121 fi 2 + N = = 11
fi N = 11 – 2 = 9 11
\ M = 2, N = 9, P = 1

Number Puzzles and Games
In this section, we will discuss some examples on number puzzles and games. These would help increase your
mathematical skills and knowledge in numbers.
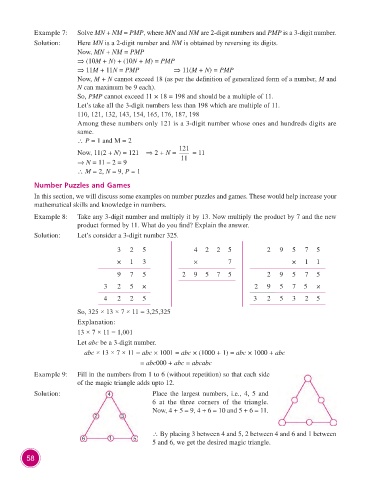
Example 8: Take any 3-digit number and multiply it by 13. Now multiply the product by 7 and the new
product formed by 11. What do you find? Explain the answer.
Solution: Let’s consider a 3-digit number 325.

3 2 5 4 2 2 5 2 9 5 7 5

× 1 3 × 7 × 1 1
9 7 5 2 9 5 7 5 2 9 5 7 5

3 2 5 × 2 9 5 7 5 ×

4 2 2 5 3 2 5 3 2 5
So, 325 × 13 × 7 × 11 = 3,25,325
Explanation:
13 × 7 × 11 = 1,001

Let abc be a 3-digit number.
abc × 13 × 7 × 11 = abc × 1001 = abc × (1000 + 1) = abc × 1000 + abc
= abc000 + abc = abcabc
Example 9: Fill in the numbers from 1 to 6 (without repetition) so that each side
of the magic triangle adds upto 12.
Solution: 4 Place the largest numbers, i.e., 4, 5 and
6 at the three corners of the triangle.
Now, 4 + 5 = 9, 4 + 6 = 10 and 5 + 6 = 11.
2 3

\ By placing 3 between 4 and 5, 2 between 4 and 6 and 1 between
6 1 5
5 and 6, we get the desired magic triangle.

58