Page 69 - ICSE Math 8

3. Form all the possible 3-digit numbers using the digits 2, 3 and 4 (without repeating the digits) which are
divisible by: (a) 2 and (b) 3.
4. Which of the given numbers are divisible by: (a) 5 and (b) 10?
35, 142, 200, 750, 109, 625, 130
5. If 31z5 is a multiple of 3, where z is a digit, then what might be the value(s) of z?
6. If a number 43y is a multiple of 9, where y is a digit, then find the value of y.
Letters for Digits

Here, we have number puzzles with letters instead of digits in an arithmetic sum and the aim is to find out
which letter represents which digit.
The two important rules for cracking the code of these puzzles are:
(1) Each digit is represented by just one letter.
(2) The first digit of the number cannot be zero.
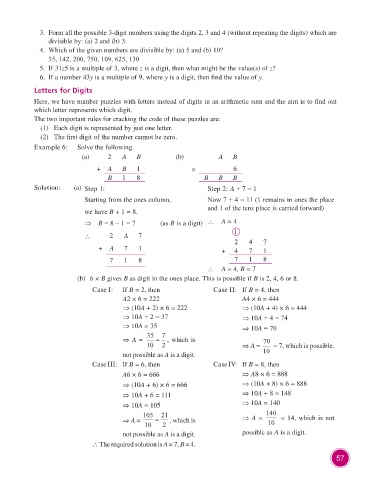
Example 6: Solve the following.
(a) 2 A B (b) A B

+ A B 1 ¥ 6
B 1 8 B B B
Solution: (a) Step 1: Step 2: A + 7 = 1

Starting from the ones column, Now 7 + 4 = 11 (1 remains in ones the place
and 1 of the tens place is carried forward)
we have B + 1 = 8.
fi B = 8 – 1 = 7 (as B is a digit) \ A = 4
1
\ 2 A 7
2 4 7
+ A 7 1 + 4 7 1

7 1 8 7 1 8
\ A = 4, B = 7
(b) 6 × B gives B as digit in the ones place. This is possible if B is 2, 4, 6 or 8.

Case I: If B = 2, then Case II: If B = 4, then
A2 × 6 = 222 A4 × 6 = 444
fi (10A + 2) × 6 = 222 fi (10A + 4) × 6 = 444
fi 10A + 2 = 37 fi 10A + 4 = 74
fi 10A = 35 fi 10A = 70
35 7
fi A = = , which is 70
10 2 fi A = = 7, which is possible.
not possible as A is a digit. 10
Case III: If B = 6, then Case IV: If B = 8, then
A6 × 6 = 666 fi A8 × 6 = 888

fi (10A + 6) × 6 = 666 fi (10A + 8) × 6 = 888
fi 10A + 6 = 111 fi 10A + 8 = 148
fi 10A = 105 fi 10A = 140
105 21 140
fi A = = , which is fi A = = 14, which is not
10 2 10
not possible as A is a digit. possible as A is a digit.
\ The required solution is A = 7, B = 4.

57