Page 48 - ICSE Math 8

8. Find the least square number exactly divisible by each one of the following numbers.
(a) 8, 9, 10 (b) 20, 15, 9, 6 (c) 4, 6, 10, 18
9. Find the square root of the following using repeated subtraction.
(a) 144 (b) 121 (c) 196 (d) 225

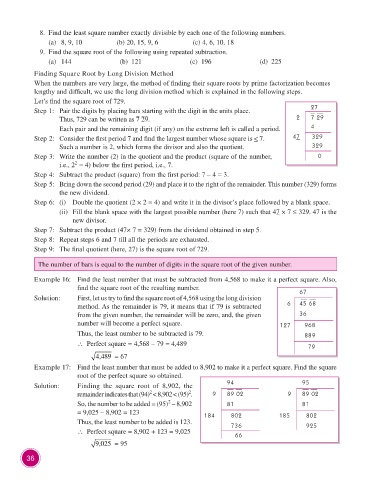
Finding Square Root by Long Division Method
When the numbers are very large, the method of finding their square roots by prime factorization becomes
lengthy and difficult, we use the long division method which is explained in the following steps.
Let’s find the square root of 729.
Step 1: Pair the digits by placing bars starting with the digit in the units place. 27
Thus, 729 can be written as 7 29. 2 7 29
Each pair and the remaining digit (if any) on the extreme left is called a period. 4
Step 2: Consider the first period 7 and find the largest number whose square is < 7. 47 329
Such a number is 2, which forms the divisor and also the quotient. 329
Step 3: Write the number (2) in the quotient and the product (square of the number, 0
2
i.e., 2 = 4) below the first period, i.e., 7.
Step 4: Subtract the product (square) from the first period: 7 – 4 = 3.
Step 5: Bring down the second period (29) and place it to the right of the remainder. This number (329) forms
the new dividend.
Step 6: (i) Double the quotient (2 × 2 = 4) and write it in the divisor’s place followed by a blank space.
(ii) Fill the blank space with the largest possible number (here 7) such that 47 × 7 ≤ 329. 47 is the
new divisor.
Step 7: Subtract the product (47× 7 = 329) from the dividend obtained in step 5.
Step 8: Repeat steps 6 and 7 till all the periods are exhausted.
Step 9: The final quotient (here, 27) is the square root of 729.

The number of bars is equal to the number of digits in the square root of the given number.

Example 16: Find the least number that must be subtracted from 4,568 to make it a perfect square. Also,
find the square root of the resulting number. 67
Solution: First, let us try to find the square root of 4,568 using the long division
method. As the remainder is 79, it means that if 79 is subtracted 6 45 68
from the given number, the remainder will be zero, and, the given 36
number will become a perfect square. 127 968
Thus, the least number to be subtracted is 79. 889
\ Perfect square = 4,568 – 79 = 4,489 79

4,489 = 67
Example 17: Find the least number that must be added to 8,902 to make it a perfect square. Find the square
root of the perfect square so obtained.
Solution: Finding the square root of 8,902, the 94 95
2
2
remainder indicates that (94) < 8,902 < (95) . 9 89 02 9 89 02
2
So, the number to be added = (95) – 8,902 81 81
= 9,025 – 8,902 = 123 184 802 185 802
Thus, the least number to be added is 123. 736 925
\ Perfect square = 8,902 + 123 = 9,025
66
9,025 = 95

36