Page 51 - ICSE Math 8

0.002025 + 0.001369 .
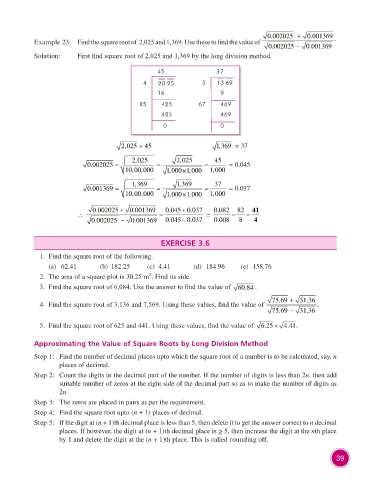
Example 23: Find the square root of 2,025 and 1,369. Use these to find the value of
0.002025 – 0.001369
Solution: First find square root of 2,025 and 1,369 by the long division method.

45 37

4 20 25 3 13 69
16 9
85 425 67 469
425 469

0 0

2,025 = 45 1,369 = 37

2,025 2,025 45
0.002025 = = = = 0.045
10,00,000 1,000×1,000 1,000

1,369 1,369 37
0.001369 = = = = 0.037
10,00,000 1,000×1,000 1,000

0.002025 + 0.001369 0.045 0.037+ 0.082 82 41
\ = = = =
−
0.002025 − 0.001369 0.045 0.037 0.008 8 4

EXERCISE 3.6

1. Find the square root of the following.
(a) 62.41 (b) 182.25 (c) 4.41 (d) 184.96 (e) 158.76
2
2. The area of a square plot is 30.25 m . Find its side.
3. Find the square root of 6,084. Use the answer to find the value of 60.84 .

75.69 + 31.36
4. Find the square root of 3,136 and 7,569. Using these values, find the value of .
75.69 − 31.36

5. Find the square root of 625 and 441. Using these values, find the value of 6.25 × 4.41.

Approximating the Value of Square Roots by Long Division Method

Step 1: Find the number of decimal places upto which the square root of a number is to be calculated, say, n
places of decimal.

Step 2: Count the digits in the decimal part of the number. If the number of digits is less than 2n, then add
suitable number of zeros at the right side of the decimal part so as to make the number of digits as
2n.
Step 3: The zeros are placed in pairs as per the requirement.
Step 4: Find the square root upto (n + 1) places of decimal.
Step 5: If the digit at (n + 1)th decimal place is less than 5, then delete it to get the answer correct to n decimal
places. If however, the digit at (n + 1)th decimal place is > 5, then increase the digit at the nth place
by 1 and delete the digit at the (n + 1)th place. This is called rounding off.

39