Page 231 - ICSE Math 8

Area of a Polygon

Area of a regular or irregular polygon can be found by using the formulae for finding the areas of a triangle,
parallelogram and trapezium. The idea is to divide the given polygon into non-overlapping rectilinear plane
figures whose areas can be found easily. The area of the polygon will be equal to the sum of the areas of the
non-overlapping parts.
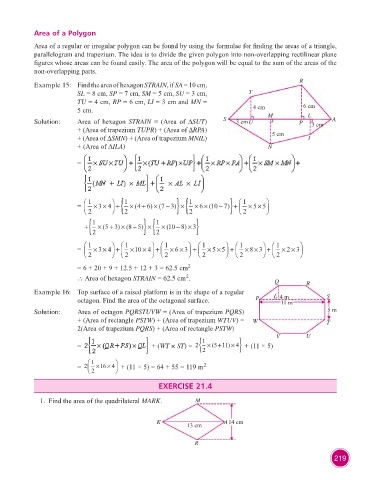
R
Example 15: Find the area of hexagon STRAIN, if SA = 10 cm,
SL = 8 cm, SP = 7 cm, SM = 5 cm, SU = 3 cm, T
TU = 4 cm, RP = 6 cm, LI = 3 cm and MN = 6 cm
5 cm. 4 cm M L

Solution: Area of hexagon STRAIN = (Area of DSUT) S 3 cm U P 3 cm A
+ (Area of trapezium TUPR) + (Area of DRPA) 5 cm
+ (Area of DSMN) + (Area of trapezium MNIL) I
+ (Area of DILA) N

=

Ê 1 ˜ { 1 } { 1 Ê 1 ˆ
ˆ
-
-
= Á 2 ¥¥ ¯ 2 ¥ ( 46 ¥) ( 73 ¥) 2 ¥ 6 ¥ ( 107 +) } Á Ë 2 ¥ 55¥ ˜ ¯
34 +
+
Ë
{ 1 } { 1 }
+ 2 ¥ 53( + ) ¥ 85( - ) ¥ 2 ¥ 10 8( - ) ¥ 3
Ê 1 ˆ Ê 1 ˆ Ê 1 ˆ Ê 1 ˆ Ê 1 ˆ Ê 1 ˆ
23
+
= Á 2 ¥¥ ˜ Á 2 ¥ 10 4¥ ˜ Á 2 ¥ 63¥ ˜ ¯ + Á 2 ¥ 55¥ ˜ ¯ + Á 2 ¥ 8 ¥ 33 + Á Ë 2 ¥ ¥ ˜ ¯
34 +
˜
¯ Ë
¯ Ë
Ë
¯
Ë
Ë
= 6 + 20 + 9 + 12.5 + 12 + 3 = 62.5 cm 2
2
\ Area of hexagon STRAIN = 62.5 cm . Q R
Example 16: Top surface of a raised platform is in the shape of a regular L S
octagon. Find the area of the octagonal surface. P 4 m
11 m
Solution: Area of octagon PQRSTUVW = (Area of trapezium PQRS) 5 m
+ (Area of rectangle PSTW) + (Area of trapezium WTUV) = W T
2(Area of trapezium PQRS) + (Area of rectangle PSTW)
{ 1 } V U
= + (WT × ST) = 2 2 ¥ ( 511+ ) ¥ 4 + (11 × 5)
Ê 1 ˆ
= 2 Á Ë 2 ¥ 16 4¥ ˜ + (11 × 5) = 64 + 55 = 119 m 2
¯

EXERCISE 21.4

1. Find the area of the quadrilateral MARK. M

K 13 cm A14 cm

R

219