Page 226 - ICSE Math 8

10. The perimeter of a rectangle is 56 m and the difference between its length and breadth is 12 m. Find its
length, breadth and area.
11. If each side of a rectangle is tripled, find the ratio between the areas of the original rectangle and the new
rectangle.
12. A rectangular field is 25 m long and 18 m wide. There are two mutually perpendicular paths each of width
3 m inside the field in such a way that one path is parallel to the length and the other path is parallel to the
breadth. Find the cost of levelling the paths at the rate of ` 12 per square metre.

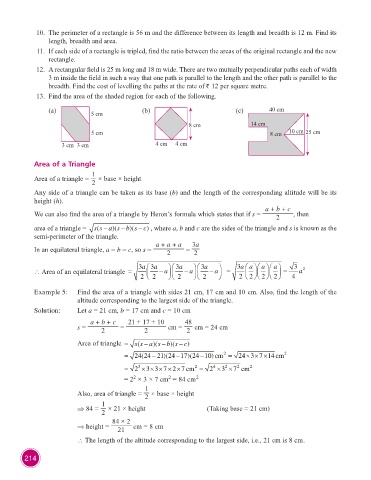
13. Find the area of the shaded region for each of the following.
(a) 5 cm (b) (c) 40 cm

8 cm 14 cm
5 cm 8 cm 10 cm 25 cm

3 cm 3 cm 4 cm 4 cm

Area of a Triangle
1
Area of a triangle = × base × height
2
Any side of a triangle can be taken as its base (b) and the length of the corresponding altitude will be its
height (h).
a + b + c
We can also find the area of a triangle by Heron’s formula which states that if s = 2 , then

area of a triangle = ss a− ( )(s b− )(s c− ) , where a, b and c are the sides of the triangle and s is known as the
semi-perimeter of the triangle.
a + a + a 3a
In an equilateral triangle, a = b = c, so s = 2 = 2

3a  3a   3a   3a  3a a a a 3 2

∴ Area of an equilateral triangle =  − a   − a   − a =  = a

2
2
2
2  2   2   2  2  4
Example 5: Find the area of a triangle with sides 21 cm, 17 cm and 10 cm. Also, find the length of the
altitude corresponding to the largest side of the triangle.
Solution: Let a = 21 cm, b = 17 cm and c = 10 cm
a + b + c 21 + 17 + 10 48
s = 2 = 2 cm = 2 cm = 24 cm

Area of triangle = ( ss a− )(s b− )(s c− )
= 24(24 21)(24 17)(24 10) cm− − − 2 = 24 3 7 14 cm×× × 2

4
2
2
3
3 3 7 2 7 cm× ×
= 2 ××× 2 = 2 × 3 × 7 cm 2
2
2
= 2 × 3 × 7 cm = 84 cm 2
1
Also, area of triangle = × base × height
2
1
⇒ 84 = × 21 × height (Taking base = 21 cm)
2
84 × 2
⇒ height = 21 cm = 8 cm
∴ The length of the altitude corresponding to the largest side, i.e., 21 cm is 8 cm.
214