Page 218 - ICSE Math 8

Example 2: In a circle of radii 6 cm, can we draw a chord of length 13 cm? Justify.
Solution: Radius of circle = 6 cm
∴ Diameter = 2 × 6 = 12 cm
Length of the chord = 13 cm > 12 cm
Since a diameter is the largest chord of a circle, therefore we cannot draw a chord of length
13 cm in a circle of radius 6 cm.
Example 3: Find the radius of a circle with centre O and diameter 18 cm. Also, find whether the points A,
B, C and D lie inside or outside or on the circle if:
(a) OA = 9 cm (b) OB = 7.5 cm (c) OC = 8 cm (d) OD = 10 cm
Solution: Diameter of circle = 18 cm 18
Diameter = 2 × Radius ⇒ 18 cm = 2 × Radius ⇒ Radius = 2 = 9 cm
(a) OA = 9 cm = r ∴ A lies on the circle.
(b) OB = 7.5 cm < r ∴ B lies inside the circle.
(c) OC = 8 cm < r ∴ C lies inside the circle.
(d) OD = 10 cm > r ∴ D lies outside the circle.

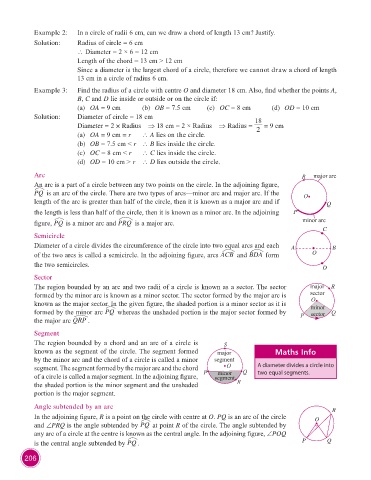
Arc
An arc is a part of a circle between any two points on the circle. In the adjoining figure,
PQ is an arc of the circle. There are two types of arcs—minor arc and major arc. If the
length of the arc is greater than half of the circle, then it is known as a major arc and if

the length is less than half of the circle, then it is known as a minor arc. In the adjoining
figure, PQ is a minor arc and PRQ is a major arc.
C
Semicircle
Diameter of a circle divides the circumference of the circle into two equal arcs and each A B
of the two arcs is called a semicircle. In the adjoining figure, arcs ACB and BDA form O
the two semicircles. D

Sector
The region bounded by an arc and two radii of a circle is known as a sector. The sector
formed by the minor arc is known as a minor sector. The sector formed by the major arc is
known as the major sector. In the given figure, the shaded portion is a minor sector as it is
formed by the minor arc PQ whereas the unshaded portion is the major sector formed by
the major arc QRP .

Segment
The region bounded by a chord and an arc of a circle is
known as the segment of the circle. The segment formed Maths Info
by the minor arc and the chord of a circle is called a minor
segment. The segment formed by the major arc and the chord A diameter divides a circle into
of a circle is called a major segment. In the adjoining figure, two equal segments.
the shaded portion is the minor segment and the unshaded
portion is the major segment.

Angle subtended by an arc R
In the adjoining figure, R is a point on the circle with centre at O. PQ is an arc of the circle O
and ∠PRQ is the angle subtended by PQ at point R of the circle. The angle subtended by
any arc of a circle at the centre is known as the central angle. In the adjoining figure, ∠POQ
is the central angle subtended by PQ . P Q

206