Page 200 - ICSE Math 8

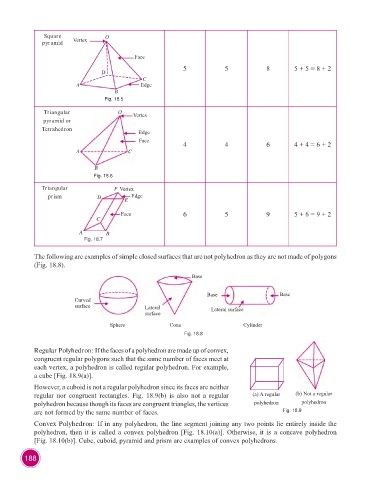
Square O
pyramid Vertex

Face
5 5 8 5 + 5 = 8 + 2
D
C
A Edge
B
Fig. 18.5

Triangular O Vertex
pyramid or
Tetrahedron
Edge
Face
4 4 6 4 + 4 = 6 + 2
A C

B
Fig. 18.6

Triangular F Vertex
prism D E Edge

Face 6 5 9 5 + 6 = 9 + 2
C

A B
Fig. 18.7

The following are examples of simple closed surfaces that are not polyhedron as they are not made of polygons
(Fig. 18.8).

Base

Base Base
Curved
surface Lateral Lateral surface
surface
Sphere Cone Cylinder
Fig. 18.8

Regular Polyhedron: If the faces of a polyhedron are made up of convex,
congruent regular polygons such that the same number of faces meet at
each vertex, a polyhedron is called regular polyhedron. For example,
a cube [Fig. 18.9(a)].
However, a cuboid is not a regular polyhedron since its faces are neither
regular nor congruent rectangles. Fig. 18.9(b) is also not a regular (a) A regular (b) Not a regular
polyhedron because though its faces are congruent triangles, the vertices polyhedron polyhedron
are not formed by the same number of faces. Fig. 18.9

Convex Polyhedron: If in any polyhedron, the line segment joining any two points lie entirely inside the
polyhedron, then it is called a convex polyhedron [Fig. 18.10(a)]. Otherwise, it is a concave polyhedron
[Fig. 18.10(b)]. Cube, cuboid, pyramid and prism are examples of convex polyhedrons.

188