Page 178 - ICSE Math 8

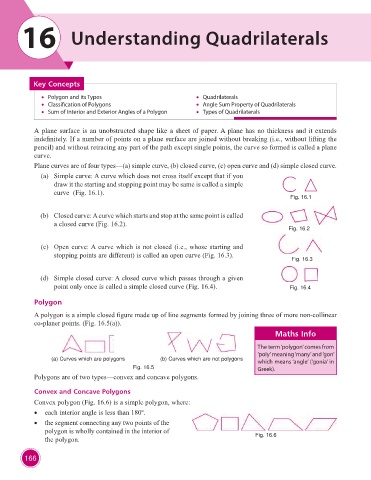
16 Understanding Quadrilaterals

Key Concepts

• Polygon and its Types • Quadrilaterals
• Classification of Polygons • Angle Sum Property of Quadrilaterals
• Sum of Interior and Exterior Angles of a Polygon • Types of Quadrilaterals

A plane surface is an unobstructed shape like a sheet of paper. A plane has no thickness and it extends
indefinitely. If a number of points on a plane surface are joined without breaking (i.e., without lifting the
pencil) and without retracing any part of the path except single points, the curve so formed is called a plane
curve.
Plane curves are of four types—(a) simple curve, (b) closed curve, (c) open curve and (d) simple closed curve.
(a) Simple curve: A curve which does not cross itself except that if you
draw it the starting and stopping point may be same is called a simple
curve (Fig. 16.1).
Fig. 16.1

(b) Closed curve: A curve which starts and stop at the same point is called
a closed curve (Fig. 16.2).
Fig. 16.2

(c) Open curve: A curve which is not closed (i.e., whose starting and
stopping points are different) is called an open curve (Fig. 16.3).
Fig. 16.3

(d) Simple closed curve: A closed curve which passes through a given
point only once is called a simple closed curve (Fig. 16.4). Fig. 16.4

Polygon

A polygon is a simple closed figure made up of line segments formed by joining three of more non-collinear
co-planer points. (Fig. 16.5(a)).
Maths Info

The term ‘polygon’ comes from
‘poly’ meaning ‘many’ and ‘gon’
(a) Curves which are polygons (b) Curves which are not polygons which means ‘angle’ (‘gonia’ in
Fig. 16.5 Greek).
Polygons are of two types—convex and concave polygons.

Convex and Concave Polygons
Convex polygon (Fig. 16.6) is a simple polygon, where:
• each interior angle is less than 180º.
• the segment connecting any two points of the
polygon is wholly contained in the interior of Fig. 16.6
the polygon.

166