Page 177 - ICSE Math 8

7. Meenakshi has a locker in a bank. She has a total of ` 2,80,000 in her locker. The currency notes are in the
denomination of ` 50, ` 20 and ` 10. These currency notes are in the ratio 3 : 4 : 5. How many notes of
each denomination are there in her locker? Is it safe to share your locker’s password with anyone? Why?
8. For a train journey, (20x + 50) passengers bought tickets costing ` 500 each and (30x – 20) passengers
bought tickets costing ` 750 each. If the train has a capacity of 480 passengers and was just full, find the
number of passengers for each category of ticket and also the total money paid for the ticket.
9. Find the solution set of the following inequations.
(a) 4(x – 3) < x + 6, x ∈ W (b) 4x – 2 ≥ 12, x ∈ {–2, –1, 0, 1, 2, 3}
5
x x 3x 1
(c) – 1 ≥ – 1, x ∈ N (d) – 1 < x + , x ∈ N
2 3 2 2
10. Find the maximum value of x from the following inequations.
1
(a) 2(x – 5) ≥ 4x – 6 (b) x – ≤ 5
3 4 12
11. Find the minimum value of x from the following inequations.
x
(a) 5x – 4 ≥ 16 (b) 3 2 + 1 + 1 ≥ 10
12. Graph the following inequalities.
(a) x ≤ 6, x ∈ Z (b) x > –1, x ∈ W
x
(c) x – 12 ≥ – 13, x ∈ R (d) x + 2 < + 3, x ∈ N
2 4

(e) 5(2x – 3) ≥ 3x – 2(x – 6), x ∈ R (f) 0 ≤ 5 – x < 5, x ∈ R
2
13. In a stage show, the standard tickets are available for ` 125 and VIP tickets are available for ` 500. The
organisers hope to collect at least ` 60,000. Select the inequaltiy that describes this situation.
Use x = the number of standard tickets and y = the number of VIP tickets
(a) 125x + 500y > 60,000 (b) 125x + 500y ≤ 60,000
(c) 125x + 500y ≥ 60,000 (d) 125x + 500y < 60,000

THINK SMART

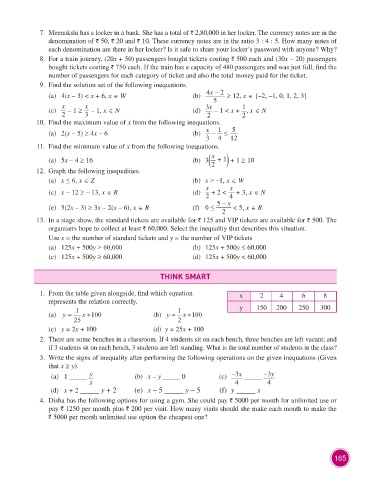
1. From the table given alongside, find which equation x 2 4 6 8
represents the relation correctly.
1 1 y 150 200 250 300
(a) y = x +100 (b) y = x +100
25 2
(c) y = 2x + 100 (d) y = 25x + 100
2. There are some benches in a classroom. If 4 students sit on each bench, three benches are left vacant; and
if 3 students sit on each bench, 3 students are left standing. What is the total number of students in the class?
3. Write the signs of inequality after performing the following operations on the given inequations (Given
that x ≥ y).
(a) 1 _____ y (b) x – y _____ 0 (c) –3x _____ –3y
x 4 4
(d) x + 2 _____ y + 2 (e) x – 5 _____ y – 5 (f) y _____ x
4. Disha has the following options for using a gym. She could pay ` 5000 per month for unlimited use or
pay ` 1250 per month plus ` 200 per visit. How many visits should she make each month to make the
` 5000 per month unlimited use option the cheapest one?

165