Page 168 - ICSE Math 8

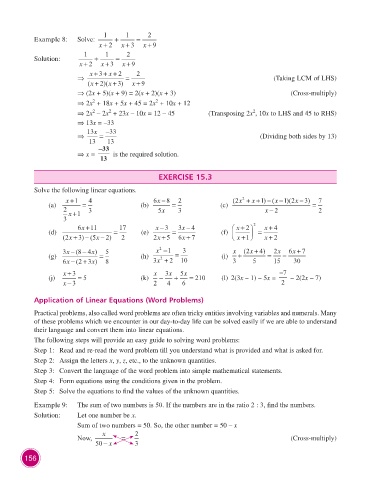
1 1 2
Example 8: Solve: + =
x + 2 x + 3 x + 9
1 1 2
Solution: + =
x + 2 x + 3 x + 9
x ++ +3 x 2 2
fi = (Taking LCM of LHS)
( x + 2)( x + 3) x + 9
fi (2x + 5)(x + 9) = 2(x + 2)(x + 3) (Cross-multiply)
2
2
fi 2x + 18x + 5x + 45 = 2x + 10x + 12
2
2
2
fi 2x – 2x + 23x – 10x = 12 – 45 (Transposing 2x , 10x to LHS and 45 to RHS)
fi 13x = –33
13x - 33
fi = (Dividing both sides by 13)
13 13
−−33
fi x = is the required solution.
13
EXERCISE 15.3

Solve the following linear equations.
2
x
)(
x +1 4 6x - 8 2 (2x ++ 1 ) (x- - 12x - ) 3 7
(a) = (b) = (c) =
2 x +1 3 5x 3 x - 2 2
3
6x + 11 17 x - 3 3 x - 4 Ê x + ˆ 2 2 x + 4
(d) = (e) = (f) Á ˜ =
( 2x + 3 -) ( 5x - 2) 2 2 x + 5 6 x + 7 Ë x + ¯ 1 x + 2
2
3x - ( 8 4x- ) 5 x - 1 3 x 2 ( x 4+ ) 2 x 6 x 7+
(g) = (h) 2 = (i) + = -
6x - ( 2 3x) 8 x 3 + 2 10 3 5 15 30
+
x + 3 x x 3 x 5 −7
(j) = 5 (k) - + = 210 (l) 2(3x – 1) – 5x = – 2(2x – 7)
x - 3 2 4 6 2

Application of Linear Equations (Word Problems)
Practical problems, also called word problems are often tricky entities involving variables and numerals. Many
of these problems which we encounter in our day-to-day life can be solved easily if we are able to understand
their language and convert them into linear equations.
The following steps will provide an easy guide to solving word problems:
Step 1: Read and re-read the word problem till you understand what is provided and what is asked for.
Step 2: Assign the letters x, y, z, etc., to the unknown quantities.
Step 3: Convert the language of the word problem into simple mathematical statements.

Step 4: Form equations using the conditions given in the problem.
Step 5: Solve the equations to find the values of the unknown quantities.

Example 9: The sum of two numbers is 50. If the numbers are in the ratio 2 : 3, find the numbers.
Solution: Let one number be x.
Sum of two numbers = 50. So, the other number = 50 – x
x 2
Now, = (Cross-multiply)
50 - x 3

156