Page 165 - ICSE Math 8

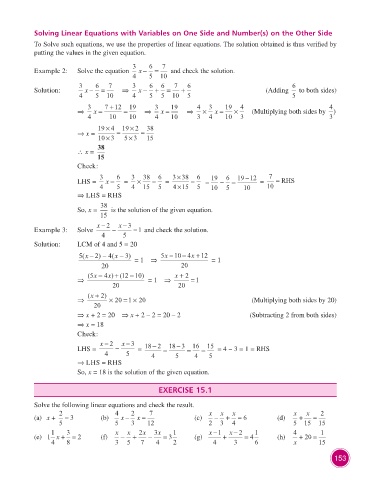
Solving Linear Equations with Variables on One Side and Number(s) on the Other Side

To Solve such equations, we use the properties of linear equations. The solution obtained is thus verified by
putting the values in the given equation.

3 6 7
Example 2: Solve the equation x - = and check the solution.
4 5 10
3 6 7 3 6 6 7 6 6
Solution: x - = fi x - + = + (Adding to both sides)
4 5 10 4 5 5 10 5 5

3 712+ 19 3 19 4 3 19 4 4
fi x = = fi x = fi ¥ x = ¥ (Multiplying both sides by )
4 10 10 4 10 3 4 10 3 3
19 4¥ 19 2¥ 38
fi x = = =
10 3¥ 53¥ 15
38
\ x =
15
Check:
3 6 3 38 6 338¥ 6 19 6 19 −12 7
LHS = x - = ¥ - = - = − = = = RHS
4 5 4 15 5 415¥ 5 10 5 10 10
fi LHS = RHS
38
So, x = is the solution of the given equation.
15
x - 2 x - 3
Example 3: Solve - =1 and check the solution.
4 5
Solution: LCM of 4 and 5 = 20

5(x − 2) − 4(x − 3) 5x - 104x- + 12
= 1 fi = 1
20 20
(5x - 4x ) (12 10+ - ) x + 2
fi = 1 fi =1
20 20
(x + 2 )
fi ¥ 20 = ¥1 20 (Multiplying both sides by 20)
20
fi x + 2 = 20 fi x + 2 – 2 = 20 – 2 (Subtracting 2 from both sides)
fi x = 18
Check:
x − 2 x −3 18 2- 18 3- 16 15
LHS = − = - = - = 4 – 3 = 1 = RHS
4 5 4 5 4 5
fi LHS = RHS
So, x = 18 is the solution of the given equation.

EXERCISE 15.1

Solve the following linear equations and check the result.
2 4 2 7 x x x x x 2
(a) x + =3 (b) x - x = (c) - + = 6 (d) + =
5 5 3 12 2 3 4 5 15 15
1 3 x x 2 x x 3 1 x -1 x - 2 1 4 1
(e) 1 x + = 2 (f) - + - = 3 (g) + = 4 (h) + 20 =
4 8 3 5 7 4 2 4 3 6 x 15

153