Page 268 - Start Up Mathematics_8 (Non CCE)

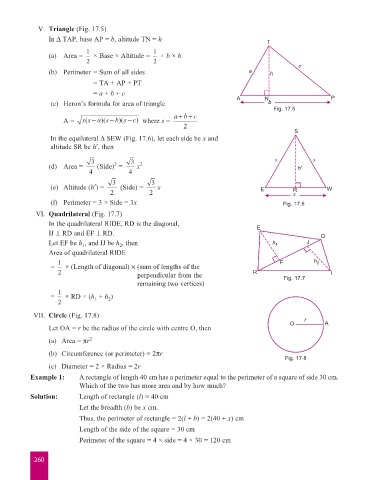
V. Triangle (Fig. 17.5)
In D TAP, base AP = b, altitude TN = h T

1 1
(a) Area = × Base × Altitude = × b × h
2 2
c
(b) Perimeter = Sum of all sides a h
= TA + AP + PT
= a + b + c P

(c) Heron’s formula for area of triangle A N b
Fig. 17.5
abc++
A = ss as bs c( - )( - )( - ) where s =
2
S
In the equilateral D SEW (Fig. 17.6), let each side be x and
altitude SR be h¢, then

3 3 x x
2
(d) Area = (Side) = x 2 h¢
4 4
3 3
(e) Altitude (h¢) = (Side) = x E W
2 2 R
x
(f) Perimeter = 3 × Side = 3x Fig. 17.6
VI. Quadrilateral (Fig. 17.7)
In the quadrilateral RIDE, RD is the diagonal, E
IJ ^ RD and EF ^ RD. D
Let EF be h , and IJ be h , then h 1 J
1
2
Area of quadrilateral RIDE
1 F h 2
= × (Length of diagonal) ¥ (sum of lengths of the
2 perpendicular from the R Fig. 17.7 I
remaining two vertices)
1
= × RD × (h + h )
1
2
2
VII. Circle (Fig. 17.8)
O r A
Let OA = r be the radius of the circle with centre O, then
(a) Area = pr 2
(b) Circumference (or perimeter) = 2pr
Fig. 17.8
(c) Diameter = 2 × Radius = 2r
Example 1: A rectangle of length 40 cm has a perimeter equal to the perimeter of a square of side 30 cm.
Which of the two has more area and by how much?
Solution: Length of rectangle (l) = 40 cm

Let the breadth (b) be x cm.
Thus, the perimeter of rectangle = 2(l + b) = 2(40 + x) cm
Length of the side of the square = 30 cm
Perimeter of the square = 4 × side = 4 × 30 = 120 cm

260