Page 267 - Start Up Mathematics_8 (Non CCE)

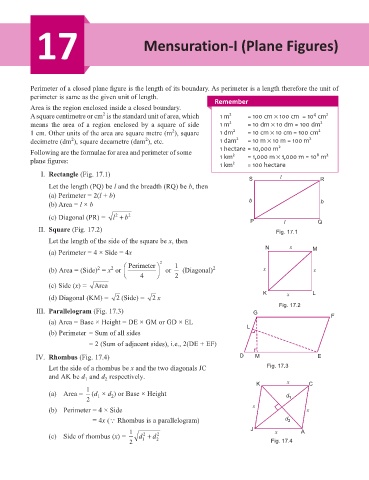
17 Mensuration-I (Plane Figures)

Perimeter of a closed plane figure is the length of its boundary. As perimeter is a length therefore the unit of
perimeter is same as the given unit of length. Remember
Area is the region enclosed inside a closed boundary.
2
4
2
A square centimetre or cm is the standard unit of area, which 1 m = 100 cm ¥ 100 cm = 10 cm 2
2
means the area of a region enclosed by a square of side 1 m = 10 dm ¥ 10 dm = 100 dm 2
2
2
1 cm. Other units of the area are square metre (m ), square 1 dm = 10 cm ¥ 10 cm = 100 cm 2
2
2
2
decimetre (dm ), square decametre (dam ), etc. 1 dam = 10 m ¥ 10 m = 100 m 2
2
Following are the formulae for area and perimeter of some 1 hectare = 10,000 m 6 2
2
1 km
= 1,000 m ¥ 1,000 m = 10 m
plane figures:
2
1 km = 100 hectare
I. Rectangle (Fig. 17.1)
S l R
Let the length (PQ) be l and the breadth (RQ) be b, then
(a) Perimeter = 2(l + b)
(b) Area = l × b b b
2
(c) Diagonal (PR) = l + b 2
P l Q
II. Square (Fig. 17.2) Fig. 17.1
Let the length of the side of the square be x, then
N x M
(a) Perimeter = 4 × Side = 4x
2
2
(b) Area = (Side) = x or Ê Perimeterˆ ˜ ¯ 2 or 1 (Diagonal) 2 x x
Á
Ë
2
4
(c) Side (x) = Area
K x L
(d) Diagonal (KM) = 2 (Side) = 2 x
Fig. 17.2
III. Parallelogram (Fig. 17.3) G F
(a) Area = Base × Height = DE × GM or GD × EL
L
(b) Perimeter = Sum of all sides
= 2 (Sum of adjacent sides), i.e., 2(DE + EF)
IV. Rhombus (Fig. 17.4) D M E

Let the side of a rhombus be x and the two diagonals JC Fig. 17.3
and AK be d and d respectively.
1
2
K x C
1
(a) Area = (d × d ) or Base × Height d
2
1
2 1
x
(b) Perimeter = 4 × Side x
= 4x ( Rhombus is a parallelogram) d 2
1 J x A
2
(c) Side of rhombus (x) = d + d 2
2 1 2 Fig. 17.4