Page 63 - ICSE Math 5

Prime Factors

Prime factors of a number are the prime numbers that divide the number exactly.

Prime factors of a number can be obtained by factor tree method and by prime factorization
method. Let’s learn to find prime factors of a number using these methods with the help of the
following examples.

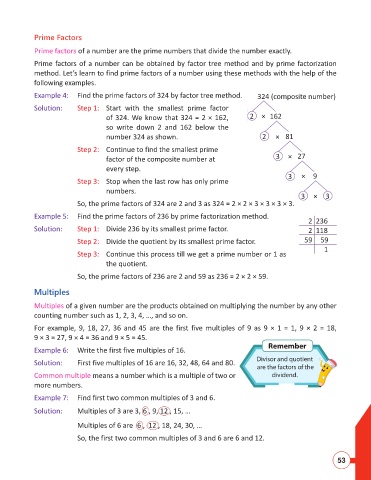
Example 4: Find the prime factors of 324 by factor tree method. 324 (composite number)
Solution: Step 1: Start with the smallest prime factor
of 324. We know that 324 = 2 × 162, 2× 162
so write down 2 and 162 below the
number 324 as shown. 2 × 81

Step 2: Continue to find the smallest prime
factor of the composite number at 3 × 27
every step.
3 × 9
Step 3: Stop when the last row has only prime
numbers.
3 × 3
So, the prime factors of 324 are 2 and 3 as 324 = 2 × 2 × 3 × 3 × 3 × 3.

Example 5: Find the prime factors of 236 by prime factorization method.
2 236
Solution: Step 1: Divide 236 by its smallest prime factor. 2 118

Step 2: Divide the quotient by its smallest prime factor. 59 59
1
Step 3: Continue this process till we get a prime number or 1 as
the quotient.
So, the prime factors of 236 are 2 and 59 as 236 = 2 × 2 × 59.

Multiples

Multiples of a given number are the products obtained on multiplying the number by any other
counting number such as 1, 2, 3, 4, …, and so on.

For example, 9, 18, 27, 36 and 45 are the first five multiples of 9 as 9 × 1 = 1, 9 × 2 = 18,
9 × 3 = 27, 9 × 4 = 36 and 9 × 5 = 45.
Remember
Example 6: Write the first five multiples of 16.
Divisor and quotient
Solution: First five multiples of 16 are 16, 32, 48, 64 and 80.
are the factors of the
Common multiple means a number which is a multiple of two or dividend.
more numbers.
Example 7: Find first two common multiples of 3 and 6.

Solution: Multiples of 3 are 3, 6 , 9, 12 , 15, …

Multiples of 6 are 6 , 12 , 18, 24, 30, …

So, the first two common multiples of 3 and 6 are 6 and 12.

53