Page 79 - Viva

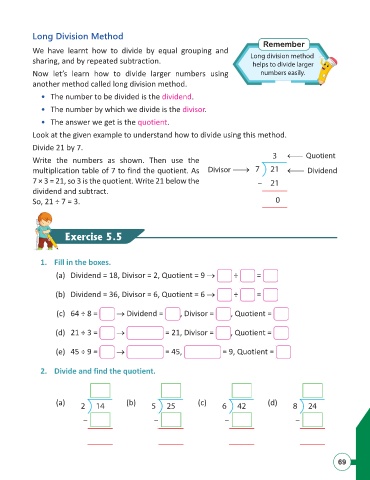
Long Division Method
Remember
We have learnt how to divide by equal grouping and
sharing, and by repeated subtraction. Long division method
helps to divide larger
Now let’s learn how to divide larger numbers using numbers easily.
another method called long division method.

• The number to be divided is the dividend.
• The number by which we divide is the divisor.

• The answer we get is the quotient.

Look at the given example to understand how to divide using this method.
Divide 21 by 7.

Write the numbers as shown. Then use the 3 ← Quotient
multiplication table of 7 to find the quotient. As Divisor → ← Dividend
7 21
7 × 3 = 21, so 3 is the quotient. Write 21 below the – 21
dividend and subtract.
So, 21 ÷ 7 = 3. 0

Exercise 5.5

1. Fill in the boxes.

(a) Dividend = 18, Divisor = 2, Quotient = 9 → ÷ =

(b) Dividend = 36, Divisor = 6, Quotient = 6 → ÷ =

(c) 64 ÷ 8 = → Dividend = , Divisor = , Quotient =

(d) 21 ÷ 3 = → = 21, Divisor = , Quotient =

(e) 45 ÷ 9 = → = 45, = 9, Quotient =

2. Divide and find the quotient.

(a) 2 14 (b) 5 25 (c) 6 42 (d) 8 24

– – – –

69