Page 41 - Start Up Mathematics_7

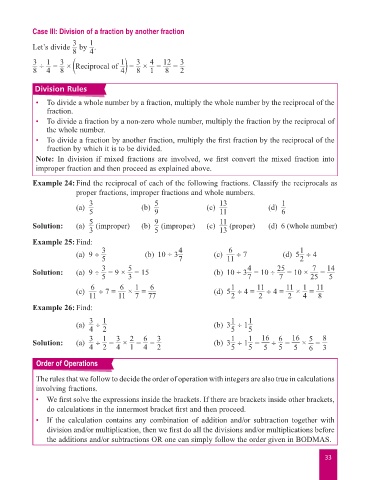
Case III: Division of a fraction by another fraction
3 1
Let’s divide by .
8 4
1
3
3 ÷ = × Reciprocal of = × = 12 = 3
3
4
1
8 4 8 4 8 1 8 2
Division Rules
• To divide a whole number by a fraction, multiply the whole number by the reciprocal of the
fraction.
• To divide a fraction by a non-zero whole number, multiply the fraction by the reciprocal of
the whole number.
• To divide a fraction by another fraction, multiply the first fraction by the reciprocal of the
fraction by which it is to be divided.
Note: In division if mixed fractions are involved, we first convert the mixed fraction into
improper fraction and then proceed as explained above.

Example 24: Find the reciprocal of each of the following fractions. Classify the reciprocals as
proper fractions, improper fractions and whole numbers.
3 5 13 1
(a) (b) (c) (d)
5 9 11 6
5 9 11
Solution: (a) (improper) (b) (improper) (c) (proper) (d) 6 (whole number)
3 5 13
Example 25: Find:
3 4 6 1
(a) 9 ÷ (b) 10 ÷ 3 (c) ÷ 7 (d) 5 ÷ 4
5 7 11 2
5
3
4
Solution: (a) 9 ÷ = 9 × = 15 (b) 10 ÷ 3 = 10 ÷ 25 = 10 × 7 = 14
5 3 7 7 25 5
6 6 1 6 1 11 11 1 11
(c) ÷ 7 = × = (d) 5 ÷ 4 = ÷ 4 = × =
11 11 7 77 2 2 2 4 8
Example 26: Find:

3 1 1 1
(a) ÷ (b) 3 ÷ 1
4 2 5 5
3
6
2
1
5
6
1
3
1
Solution: (a) 3 ÷ = × = = (b) 3 ÷ 1 = 16 ÷ = 16 × = 8
4 2 4 1 4 2 5 5 5 5 5 6 3
Order of Operations
The rules that we follow to decide the order of operation with integers are also true in calculations
involving fractions.
• We first solve the expressions inside the brackets. If there are brackets inside other brackets,
do calculations in the innermost bracket first and then proceed.
• If the calculation contains any combination of addition and/or subtraction together with
division and/or multiplication, then we first do all the divisions and/or multiplications before
the additions and/or subtractions OR one can simply follow the order given in BODMAS.

33