Page 25 - Start Up Mathematics_7

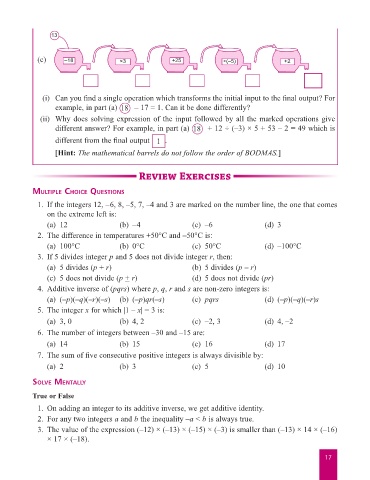
13

(c) –18 ×3 +25 ÷(–5) +2

(i) Can you find a single operation which transforms the initial input to the final output? For
example, in part (a) 18 – 17 = 1. Can it be done differently?
(ii) Why does solving expression of the input followed by all the marked operations give
different answer? For example, in part (a) 18 + 12 ÷ (–3) × 5 + 53 – 2 = 49 which is

different from the final output 1 .
[Hint: The mathematical barrels do not follow the order of BODMAS.]

Review Exercises

Multiple ChoiCe Questions
1. If the integers 12, –6, 8, –5, 7, –4 and 3 are marked on the number line, the one that comes
on the extreme left is:
(a) 12 (b) –4 (c) –6 (d) 3
2. The difference in temperatures +50°C and –50°C is:

(a) 100°C (b) 0°C (c) 50°C (d) –100°C
3. If 5 divides integer p and 5 does not divide integer r, then:
(a) 5 divides (p + r) (b) 5 divides (p – r)
(c) 5 does not divide (p + r) (d) 5 does not divide (pr)
4. Additive inverse of (pqrs) where p, q, r and s are non-zero integers is:

(a) (–p)(–q)(–r)(–s) (b) (–p)qr(–s) (c) pqrs (d) (–p)(–q)(–r)s
5. The integer x for which |1 – x| = 3 is:
(a) 3, 0 (b) 4, 2 (c) –2, 3 (d) 4, –2

6. The number of integers between –30 and –15 are:
(a) 14 (b) 15 (c) 16 (d) 17
7. The sum of five consecutive positive integers is always divisible by:
(a) 2 (b) 3 (c) 5 (d) 10

solve Mentally

True or False
1. On adding an integer to its additive inverse, we get additive identity.
2. For any two integers a and b the inequality –a < b is always true.

3. The value of the expression (–12) × (–13) × (–15) × (–3) is smaller than (–13) × 14 × (–16)
× 17 × (–18).

17