Page 240 - Start Up Mathematics_7

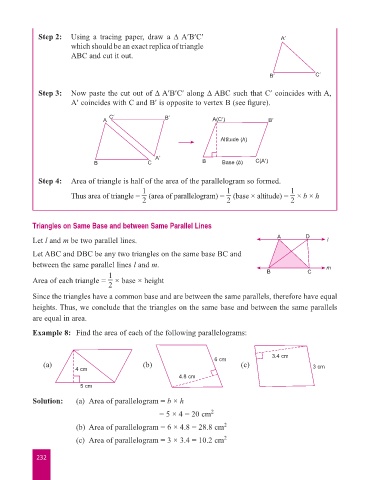
Step 2: Using a tracing paper, draw a ∆ A′B′C′ A′
which should be an exact replica of triangle
ABC and cut it out.

B′ C′

Step 3: Now paste the cut out of ∆ A′B′C′ along ∆ ABC such that C′ coincides with A,
A′ coincides with C and B′ is opposite to vertex B (see figure).

C′ B′
A A(C′) B′

Altitude (h)

A′
B C B Base (b) C(A′)

Step 4: Area of triangle is half of the area of the parallelogram so formed.
1 1 1
Thus area of triangle = (area of parallelogram) = (base × altitude) = × b × h
2 2 2

Triangles on Same Base and between Same Parallel Lines
Let l and m be two parallel lines. A D l

Let ABC and DBC be any two triangles on the same base BC and

between the same parallel lines l and m. m
1 B C
Area of each triangle = × base × height
2
Since the triangles have a common base and are between the same parallels, therefore have equal
heights. Thus, we conclude that the triangles on the same base and between the same parallels
are equal in area.

Example 8: Find the area of each of the following parallelograms:

3.4 cm
6 cm
(a) (b) (c) 3 cm
4 cm
4.8 cm
5 cm

Solution: (a) Area of parallelogram = b × h
= 5 × 4 = 20 cm 2

(b) Area of parallelogram = 6 × 4.8 = 28.8 cm 2

(c) Area of parallelogram = 3 × 3.4 = 10.2 cm 2

232