Page 228 - Start Up Mathematics_6

Dividing a Number in the Given Ratio
If the number p is to be divided in the ratio a : b, then
a b
first part = c m × p and the second part = c m × p.
a + b a + b
For example, let’s divide 35 in the ratio 2 : 3.
2 2 3 3
First part = c 2 + 3 m× 35 = 5 × 35 = 14; second part = c 2 + 3 m × 35 = 5 × 35 = 21

Comparison of Ratios
To compare two or more ratios, we first convert them into fractions and then follow the method
learnt in the chapter on fractions.
Example 1: There are 20 girls and 25 boys in a class.
(a) What is the ratio of number of girls to the number of boys?
(b) What is the ratio of number of girls to the total number of students in the class?

Solution: (a) Ratio of number of girls to the number of boys = 20 : 25 = 4 : 5
(b) Ratio of number of girls to the total number of students in the class
= 20 : (20 + 25) = 20 : 45 = 4 : 9
Example 2: Out of 40 students in a class, 9 like football, 15 like cricket and the remaining like
tennis. Find the ratio of:

(a) number of students liking cricket to the number of students liking tennis.
(b) number of students liking cricket to the total number of students.
Solution: Total number of students in the class = 40

Number of students who like tennis = 40 – (9 + 15) = 16
(a) Number of students liking cricket to tennis = 15 : 16

(b) Number of students liking cricket to total number of students = 15 : 40 = 3 : 8
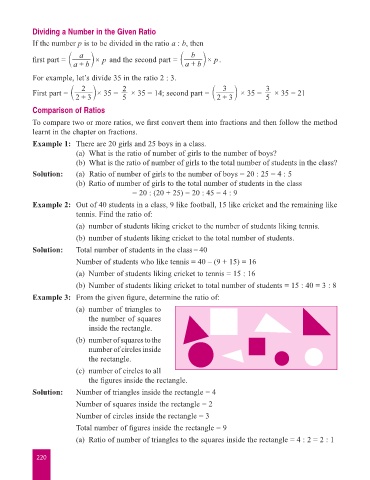
Example 3: From the given figure, determine the ratio of:

(a) number of triangles to
the number of squares
inside the rectangle.

(b) number of squares to the
number of circles inside
the rectangle.
(c) number of circles to all
the figures inside the rectangle.

Solution: Number of triangles inside the rectangle = 4
Number of squares inside the rectangle = 2

Number of circles inside the rectangle = 3
Total number of figures inside the rectangle = 9

(a) Ratio of number of triangles to the squares inside the rectangle = 4 : 2 = 2 : 1

220