Page 117 - Start Up Mathematics_6

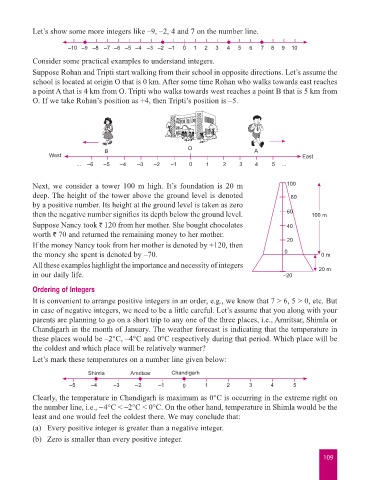
Let’s show some more integers like –9, –2, 4 and 7 on the number line.

–10 –9 –8 –7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7 8 9 10

Consider some practical examples to understand integers.
Suppose Rohan and Tripti start walking from their school in opposite directions. Let’s assume the
school is located at origin O that is 0 km. After some time Rohan who walks towards east reaches
a point A that is 4 km from O. Tripti who walks towards west reaches a point B that is 5 km from
O. If we take Rohan’s position as +4, then Tripti’s position is –5.

B O A
West East
... –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 ...

Next, we consider a tower 100 m high. It’s foundation is 20 m 100
deep. The height of the tower above the ground level is denoted 80
by a positive number. Its height at the ground level is taken as zero
then the negative number signifies its depth below the ground level. 60 100 m
Suppose Nancy took ` 120 from her mother. She bought chocolates 40
worth ` 70 and returned the remaining money to her mother.
If the money Nancy took from her mother is denoted by +120, then 20
the money she spent is denoted by –70. 0 0 m

All these examples highlight the importance and necessity of integers 20 m
in our daily life. –20

Ordering of Integers
It is convenient to arrange positive integers in an order, e.g., we know that 7 > 6, 5 > 0, etc. But
in case of negative integers, we need to be a little careful. Let’s assume that you along with your
parents are planning to go on a short trip to any one of the three places, i.e., Amritsar, Shimla or
Chandigarh in the month of January. The weather forecast is indicating that the temperature in
these places would be –2°C, –4°C and 0°C respectively during that period. Which place will be
the coldest and which place will be relatively warmer?
Let’s mark these temperatures on a number line given below:

Shimla Amritsar Chandigarh

–5 –4 –3 –2 –1 0 1 2 3 4 5
Clearly, the temperature in Chandigarh is maximum as 0°C is occurring in the extreme right on
the number line, i.e., –4°C < –2°C < 0°C. On the other hand, temperature in Shimla would be the
least and one would feel the coldest there. We may conclude that:
(a) Every positive integer is greater than a negative integer.

(b) Zero is smaller than every positive integer.

109