Page 86 - Start Up Mathematics_5

3. Multiply the given numbers.

5 3 1 1 3 18 15
(a) 1 × 3 (b) 2 × 2 (c) 3 × 5 (d) 2 × 1 (e) ×
7 5 2 4 5 5 14
1 5 6 55 4 3 5 28 1
(f) 4 × 9 (g) 11 × 18 (h) 7 × 35 (i) 6 × 2 3 (j) 27 × 2 4
5
5
4. Fill in the blanks.
51 _______ 8 3 _______ 8
(a) × 0 = (b) × = ×
64 5 7 5
2 _____ 5 2 1 5 75 _______
(c) × × = × × (d) 1 × =
7 8 7 3 8 93

(e) _______ × 9 = 9 × 6 (f) _______ × 98 = 98 × 5
11 11 13 101 101 7
Reciprocal of a Fraction

Reciprocal of a fraction means inverting the given fraction, i.e., changing the numerator to
the denominator and the denominator to the numerator. It is also called the multiplicative
inverse of a fraction.

For example, reciprocal of 5 (Numerator) = 7 (Numerator) Remember
7 (Denominator) 5 (Denominator) The product of a number
16 23
Similarly, reciprocal of = . and its reciprocal is always 1.
23 16 4 5
The reciprocal of a whole number is 1 divided by the For example, 5 × 4 = 1.
whole number.
1
For example, reciprocal of 5 =
5
A mixed number is changed to an improper fraction before finding its reciprocal.
1 7 3
For example, 2 = and its reciprocal is .
3 3 7
Division of Fractions

Division of a whole number by a fraction

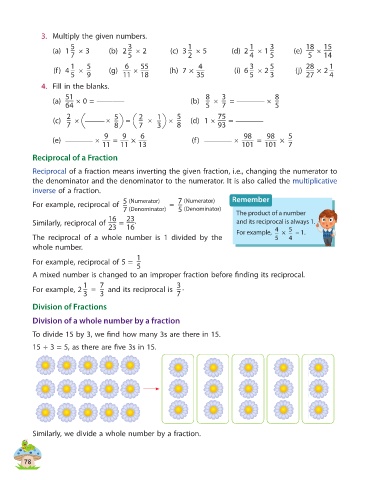
To divide 15 by 3, we find how many 3s are there in 15.
15 ÷ 3 = 5, as there are five 3s in 15.

Similarly, we divide a whole number by a fraction.

78