Page 84 - Start Up Mathematics_5

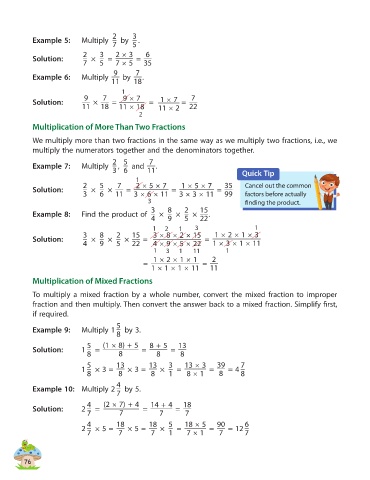
2 3
Example 5: Multiply by .
7 5
2 3 2 × 3 6
Solution: × = =
7 5 7 × 5 35
9 7
Example 6: Multiply by .
11 18
1
9 7 9 × 7 1 × 7 7
Solution: × = = =
11 18 11 × 18 11 × 2 22
2
Multiplication of More Than Two Fractions

We multiply more than two fractions in the same way as we multiply two fractions, i.e., we
multiply the numerators together and the denominators together.
2 5 7
Example 7: Multiply , and .
3 6 11 Quick Tip
1
2 5 7 2 × 5 × 7 1 × 5 × 7 35 Cancel out the common
Solution: × × = = =
3 6 11 3 × 6 × 11 3 × 3 × 11 99 factors before actually
3 finding the product.
3 8 2 15
Example 8: Find the product of × × × .
4 9 5 22
1 2 1 3 1
3 8 2 15 3 × 8 × 2 × 15 1 × 2 × 1 × 3
Solution: × × × = =
4 9 5 22 4 × 9 × 5 × 22 1 × 3 × 1 × 11
1 3 1 11 1
1 × 2 × 1 × 1 2
= =
1 × 1 × 1 × 11 11

Multiplication of Mixed Fractions

To multiply a mixed fraction by a whole number, convert the mixed fraction to improper
fraction and then multiply. Then convert the answer back to a mixed fraction. Simplify first,
if required.
5
Example 9: Multiply 1 by 3.
8
5 (1 × 8) + 5 8 + 5 13
Solution: 1 = = =
8 8 8 8
5 13 13 3 13 × 3 39 7
1 × 3 = × 3 = × = = = 4
8 8 8 1 8 × 1 8 8
4
Example 10: Multiply 2 by 5.
7
4
Solution: 2 = (2 × 7) + 4 = 14 + 4 = 18
7 7 7 7
4 18 18 5 18 × 5 90 6
2 × 5 = × 5 = × = = = 12
7 7 7 1 7 × 1 7 7

76