Page 163 - Start Up Mathematics_5

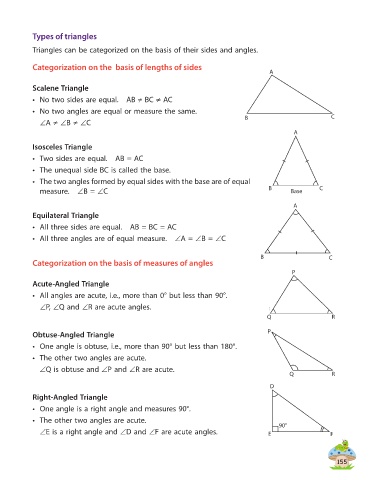
Types of triangles

Triangles can be categorized on the basis of their sides and angles.

Categorization on the basis of lengths of sides
A

Scalene Triangle

• No two sides are equal. AB ≠ BC ≠ AC
• No two angles are equal or measure the same.
∠A ≠ ∠B ≠ ∠C B C
A

Isosceles Triangle

• Two sides are equal. AB = AC
• The unequal side BC is called the base.
• The two angles formed by equal sides with the base are of equal
measure. ∠B = ∠C B Base C

A
Equilateral Triangle

• All three sides are equal. AB = BC = AC
• All three angles are of equal measure. ∠A = ∠B = ∠C

B C
Categorization on the basis of measures of angles
P

Acute-Angled Triangle
• All angles are acute, i.e., more than 0° but less than 90°.
∠P, ∠Q and ∠R are acute angles.

Q R

Obtuse-Angled Triangle P
• One angle is obtuse, i.e., more than 90° but less than 180°.

• The other two angles are acute.
∠Q is obtuse and ∠P and ∠R are acute.
Q R

D
Right-Angled Triangle
• One angle is a right angle and measures 90°.

• The other two angles are acute.
90°
∠E is a right angle and ∠D and ∠F are acute angles. E F

155