Page 61 - ICSE Math 8

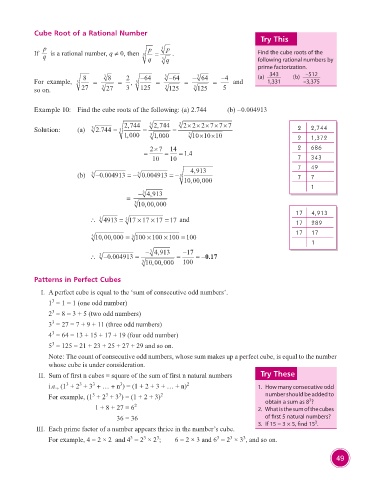
Cube Root of a Rational Number
Try This
p p 3 p
If q is a rational number, q π 0, then 3 q = 3 q . Find the cube roots of the
following rational numbers by
prime factorization.
8 3 8 2 - 64 3 - 64 - 3 64 - 4 (a) 343 (b) –512
For example, 3 = = , 3 = = = and 1,331 –3,375
so on. 27 3 27 3 125 3 125 3 125 5

Example 10: Find the cube roots of the following: (a) 2.744 (b) –0.004913

×××××
2 744, 3 2 744, 3 222 777
Solution: (a) 3 2 744. = 3 = = 2 2,744
1 000, 3 1 000, 3 10 10 10 2 1,372
×
×
×
27 14 2 686
= = =14 .
10 10 7 343
7 49
4 913,
(b) 3 -0 004913. = - 0 004913. = - 3 7 7
3
10 00 000, ,
1
3
− 4 913,
=
3 10 00 000, ,
17 4,913
3
\ 4913 = 3 17 17 17¥ ¥ = 17 and 17 289
17 17
3 10 00 000, , = 3 100 100 100¥ ¥ = 100
1
- 4 913, -17
3
\ -0 004913. = = =-0.17
3
3 10 00 000, , 100
Patterns in Perfect Cubes
I. A perfect cube is equal to the ‘sum of consecutive odd numbers’.
3
1 = 1 = 1 (one odd number)
3
2 = 8 = 3 + 5 (two odd numbers)
3
3 = 27 = 7 + 9 + 11 (three odd numbers)
3
4 = 64 = 13 + 15 + 17 + 19 (four odd number)
3
5 = 125 = 21 + 23 + 25 + 27 + 29 and so on.
Note: The count of consecutive odd numbers, whose sum makes up a perfect cube, is equal to the number
whose cube is under consideration.

II. Sum of first n cubes = square of the sum of first n natural numbers Try These
3
3
3
3
i.e., (1 + 2 + 3 + … + n ) = (1 + 2 + 3 + … + n) 2 1. How many consecutive odd
3
3
3
For example, (1 + 2 + 3 ) = (1 + 2 + 3) 2 number should be added to
3
obtain a sum as 8 ?
1 + 8 + 27 = 6 2 2. What is the sum of the cubes
36 = 36 of first 5 natural numbers?
3
III. Each prime factor of a number appears thrice in the number’s cube. 3. If 15 = 3 × 5, find 15 .
3
3
3
3
3
3
For example, 4 = 2 × 2 and 4 = 2 × 2 ; 6 = 2 × 3 and 6 = 2 × 3 , and so on.
49