Page 58 - ICSE Math 8

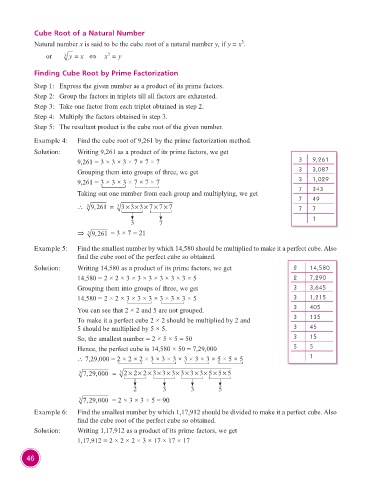
Cube Root of a Natural Number
3
Natural number x is said to be the cube root of a natural number y, if y = x .
3
or 3 y = x ¤ x = y

Finding Cube Root by Prime Factorization
Step 1: Express the given number as a product of its prime factors.

Step 2: Group the factors in triplets till all factors are exhausted.
Step 3: Take one factor from each triplet obtained in step 2.
Step 4: Multiply the factors obtained in step 3.
Step 5: The resultant product is the cube root of the given number.

Example 4: Find the cube root of 9,261 by the prime factorization method.
Solution: Writing 9,261 as a product of its prime factors, we get
9,261 = 3 × 3 × 3 × 7 × 7 × 7 3 9,261
Grouping them into groups of three, we get 3 3,087
9,261 = 3 × 3 × 3 × 7 × 7 × 7 3 1,029
7 343
Taking out one number from each group and multiplying, we get
7 49
\ 9 261, = 33 3 777×× ××× 7 7
3
3
1
3 7
fi 9 261, = 3 × 7 = 21
3
Example 5: Find the smallest number by which 14,580 should be multiplied to make it a perfect cube. Also
find the cube root of the perfect cube so obtained.
Solution: Writing 14,580 as a product of its prime factors, we get 2 14,580
14,580 = 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3 × 5 2 7,290
Grouping them into groups of three, we get 3 3,645

14,580 = 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3 × 5 3 1,215
You can see that 2 × 2 and 5 are not grouped. 3 405
To make it a perfect cube 2 × 2 should be multiplied by 2 and 3 135
5 should be multiplied by 5 × 5. 3 45
So, the smallest number = 2 × 5 × 5 = 50 3 15
Hence, the perfect cube is 14,580 × 50 = 7,29,000 5 5
\ 7,29,000 = 2 × 2 × 2 × 3 × 3 × 3 × 3 × 3 × 3 × 5 × 5 × 5 1

3 729 000, , = 22 2 333 333 555×× ××× ××× ×××
3
2 3 3 5

3 729 000, , = 2 × 3 × 3 × 5 = 90
Example 6: Find the smallest number by which 1,17,912 should be divided to make it a perfect cube. Also
find the cube root of the perfect cube so obtained.
Solution: Writing 1,17,912 as a product of its prime factors, we get
1,17,912 = 2 × 2 × 2 × 3 × 17 × 17 × 17

46