Page 244 - ICSE Math 8

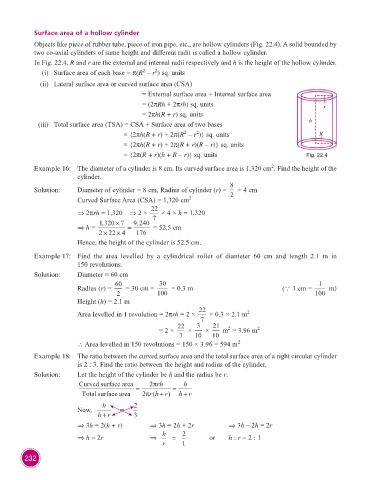
Surface area of a hollow cylinder

Objects like piece of rubber tube, piece of iron pipe, etc., are hollow cylinders (Fig. 22.4). A solid bounded by
two co-axial cylinders of same height and different radii is called a hollow cylinder.
In Fig. 22.4, R and r are the external and internal radii respectively and h is the height of the hollow cylinder.
2
2
(i) Surface area of each base = p(R – r ) sq. units
(ii) Lateral surface area or curved surface area (CSA)
= External surface area + Internal surface area
= (2pRh + 2prh) sq. units r
= 2ph(R + r) sq. units
(iii) Total surface area (TSA) = CSA + Surface area of two bases h
2
2
= {2ph(R + r) + 2p(R – r )} sq. units R
= {2ph(R + r) + 2p(R + r)(R – r)} sq. units
= {2p(R + r)(h + R – r)} sq. units Fig. 22.4
2
Example 16: The diameter of a cylinder is 8 cm. Its curved surface area is 1,320 cm . Find the height of the
cylinder.
8
Solution: Diameter of cylinder = 8 cm, Radius of cylinder (r) = 2 = 4 cm
Curved Surface Area (CSA) = 1,320 cm 2
22
fi 2prh = 1,320 fi 2 × × 4 × h = 1,320
1 320 7, ¥ 9 240, 7
fi h = = = 52.5 cm
2224¥ ¥ 176
Hence, the height of the cylinder is 52.5 cm.

Example 17: Find the area levelled by a cylindrical roller of diameter 60 cm and length 2.1 m in
150 revolutions.
Solution: Diameter = 60 cm
60 30 1
Radius (r) = = 30 cm = = 0.3 m ( 1 cm = m)
2 100 100
Height (h) = 2.1 m
22
Area levelled in 1 revolution = 2prh = 2 × 7 × 0.3 × 2.1 m 2
22 3 21
2
= 2 × × × m = 3.96 m 2
7 10 10
\ Area levelled in 150 revolutions = 150 × 3.96 = 594 m 2

Example 18: The ratio between the curved surface area and the total surface area of a right circular cylinder
is 2 : 3. Find the ratio between the height and radius of the cylinder.
Solution: Let the height of the cylinder be h and the radius be r.
Curved surface area = 2prh = h
+
Totalsurface area 2prh( + r) hr
h 2
Now, =
hr+ 3
fi 3h = 2(h + r) fi 3h = 2h + 2r fi 3h – 2h = 2r
h 2
fi h = 2r fi = or h : r = 2 : 1
r 1

232