Page 239 - ICSE Math 8

fi In 1 minute, volume of water filled = 60 L
\ In 1 h (i.e., 60 minutes), volume of water filled = 60 × 60 = 3,600 L

Now 3,600 L water is filled in 1 h
1
fi 1 L water is filled in h
3 600,
Ê 1 ˆ
fi 1,08,000 L water is filled in Á Ë 3 600, ¥ 108 000, , ˜ h = 30 h
¯
Hence, the reservoir will be filled in 30 hours.

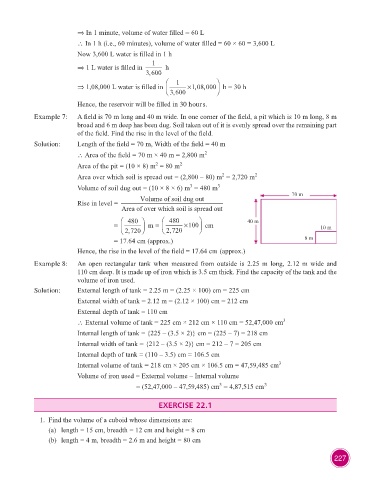
Example 7: A field is 70 m long and 40 m wide. In one corner of the field, a pit which is 10 m long, 8 m
broad and 6 m deep has been dug. Soil taken out of it is evenly spread over the remaining part
of the field. Find the rise in the level of the field.
Solution: Length of the field = 70 m, Width of the field = 40 m

\ Area of the field = 70 m × 40 m = 2,800 m 2
2
Area of the pit = (10 × 8) m = 80 m 2
2
Area over which soil is spread out = (2,800 – 80) m = 2,720 m 2
3
Volume of soil dug out = (10 × 8 × 6) m = 480 m 3
70 m
Volume of soil dug out
Rise in level =
Area of over which soil is spread outt

Ê 480 ˆ Ê 480 ˆ 40 m
= Á 2 720, ˜ ¯ m = Á Ë 2 720, ¥ 100 cm 10 m
˜
¯
Ë
= 17.64 cm (approx.) 8 m
Hence, the rise in the level of the field = 17.64 cm (approx.)
Example 8: An open rectangular tank when measured from outside is 2.25 m long, 2.12 m wide and
110 cm deep. It is made up of iron which is 3.5 cm thick. Find the capacity of the tank and the
volume of iron used.
Solution: External length of tank = 2.25 m = (2.25 × 100) cm = 225 cm
External width of tank = 2.12 m = (2.12 × 100) cm = 212 cm
External depth of tank = 110 cm
\ External volume of tank = 225 cm × 212 cm × 110 cm = 52,47,000 cm 3

Internal length of tank = {225 – (3.5 × 2)} cm = (225 – 7) = 218 cm
Internal width of tank = {212 – (3.5 × 2)} cm = 212 – 7 = 205 cm
Internal depth of tank = (110 – 3.5) cm = 106.5 cm
Internal volume of tank = 218 cm × 205 cm × 106.5 cm = 47,59,485 cm 3

Volume of iron used = External volume – Internal volume
3
= (52,47,000 – 47,59,485) cm = 4,87,515 cm 3

EXERCISE 22.1

1. Find the volume of a cuboid whose dimensions are:
(a) length = 15 cm, breadth = 12 cm and height = 8 cm
(b) length = 4 m, breadth = 2.6 m and height = 80 cm

227