Page 157 - Start Up Mathematics_8 (Non CCE)

If two quantities x and y vary inversely and x and y are its values at one point, then
1
1
x y = k, a constant ...(1)
1
1
If x and y are the values which x and y assume at second point, then
2
2
x y = k, a constant ...(2)
2
2
Equating (1) and (2), we get x y = x y 2
1
2
1
x y
fi 1 = 2
x 2 y 1
fi x : x : : y : y 1
1
2
2
fi x : x = y : y 1
1
2
2
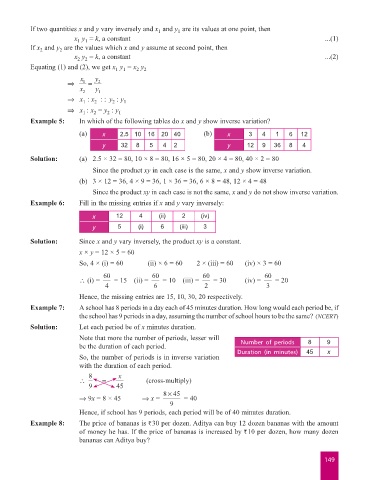
Example 5: In which of the following tables do x and y show inverse variation?
(a) x 2.5 10 16 20 40 (b) x 3 4 1 6 12
y 32 8 5 4 2 y 12 9 36 8 4
Solution: (a) 2.5 × 32 = 80, 10 × 8 = 80, 16 × 5 = 80, 20 × 4 = 80, 40 × 2 = 80
Since the product xy in each case is the same, x and y show inverse variation.
(b) 3 × 12 = 36, 4 × 9 = 36, 1 × 36 = 36, 6 × 8 = 48, 12 × 4 = 48
Since the product xy in each case is not the same, x and y do not show inverse variation.
Example 6: Fill in the missing entries if x and y vary inversely:

x 12 4 (ii) 2 (iv)
y 5 (i) 6 (iii) 3

Solution: Since x and y vary inversely, the product xy is a constant.
x × y = 12 × 5 = 60
So, 4 × (i) = 60 (ii) × 6 = 60 2 × (iii) = 60 (iv) × 3 = 60

60 60 60 60
\ (i) = = 15 (ii) = = 10 (iii) = = 30 (iv) = = 20
4 6 2 3
Hence, the missing entries are 15, 10, 30, 20 respectively.
Example 7: A school has 8 periods in a day each of 45 minutes duration. How long would each period be, if
the school has 9 periods in a day, assuming the number of school hours to be the same? (NCERT)
Solution: Let each period be of x minutes duration.
Note that more the number of periods, lesser will Number of periods 8 9
be the duration of each period.
Duration (in minutes) 45 x
So, the number of periods is in inverse variation
with the duration of each period.
8 x
\ = (cross-multiply)
9 45
845¥
fi 9x = 8 × 45 fi x = = 40
9
Hence, if school has 9 periods, each period will be of 40 minutes duration.

Example 8: The price of bananas is ` 30 per dozen. Aditya can buy 12 dozen bananas with the amount
of money he has. If the price of bananas is increased by ` 10 per dozen, how many dozen
bananas can Aditya buy?

149