Page 15 - Start Up Mathematics_7
P. 15
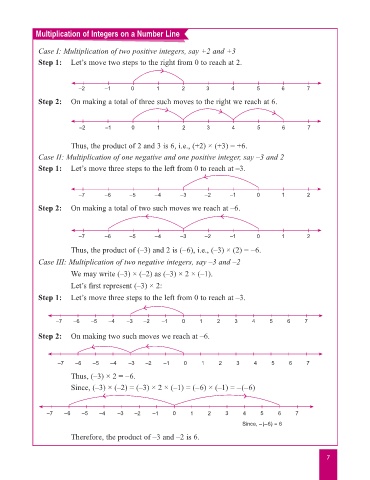
Multiplication of Integers on a Number Line
Case I: Multiplication of two positive integers, say +2 and +3
Step 1: Let’s move two steps to the right from 0 to reach at 2.
–2 –1 0 1 2 3 4 5 6 7
Step 2: On making a total of three such moves to the right we reach at 6.
–2 –1 0 1 2 3 4 5 6 7
Thus, the product of 2 and 3 is 6, i.e., (+2) × (+3) = +6.
Case II: Multiplication of one negative and one positive integer, say –3 and 2
Step 1: Let’s move three steps to the left from 0 to reach at –3.
–7 –6 –5 –4 –3 –2 –1 0 1 2
Step 2: On making a total of two such moves we reach at –6.
–7 –6 –5 –4 –3 –2 –1 0 1 2
Thus, the product of (–3) and 2 is (–6), i.e., (–3) × (2) = –6.
Case III: Multiplication of two negative integers, say –3 and –2
We may write (–3) × (–2) as (–3) × 2 × (–1).
Let’s first represent (–3) × 2:
Step 1: Let’s move three steps to the left from 0 to reach at –3.
–7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7
Step 2: On making two such moves we reach at –6.
–7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7
Thus, (–3) × 2 = –6.
Since, (–3) × (–2) = (–3) × 2 × (–1) = (–6) × (–1) = –(–6)
–7 –6 –5 –4 –3 –2 –1 0 1 2 3 4 5 6 7
Since, –(–6) = 6
Therefore, the product of –3 and –2 is 6.
7