Page 106 - Start Up Mathematics_7

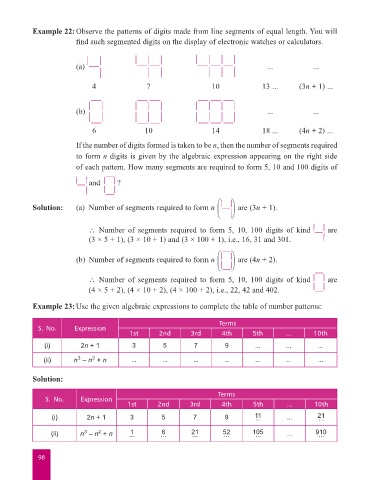
Example 22: Observe the patterns of digits made from line segments of equal length. You will

find such segmented digits on the display of electronic watches or calculators.

(a) ... ...

4 7 10 13 ... (3n + 1) ...

(b) ... ...

6 10 14 18 ... (4n + 2) ...

If the number of digits formed is taken to be n, then the number of segments required
to form n digits is given by the algebraic expression appearing on the right side
of each pattern. How many segments are required to form 5, 10 and 100 digits of

and ?

 a 
6
Solution: (a) Number of segments required to form n   are (3n + 1).
4
 a 
∴ Number of segments required to form 5, 10, 100 digits of kind are
(3 × 5 + 1), (3 × 10 + 1) and (3 × 100 + 1), i.e., 16, 31 and 301.

6
 a 
(b) Number of segments required to form n   are (4n + 2).
 a 
4
∴ Number of segments required to form 5, 10, 100 digits of kind are
(4 × 5 + 2), (4 × 10 + 2), (4 × 100 + 2), i.e., 22, 42 and 402.

Example 23: Use the given algebraic expressions to complete the table of number patterns:

Terms
S. No. Expression
1st 2nd 3rd 4th 5th ... 10th
(i) 2n + 1 3 5 7 9 ... ... ...

3
2
(ii) n – n + n ... ... ... ... ... ... ...
Solution:

Terms
S. No. Expression
1st 2nd 3rd 4th 5th ... 10th
(i) 2n + 1 3 5 7 9 11 ... 21
...
...
6
1
3
2
(ii) n – n + n ... ... 21 52 105 ... 910
...
...
...
...
98