Page 274 - Start Up Mathematics_6

Section D
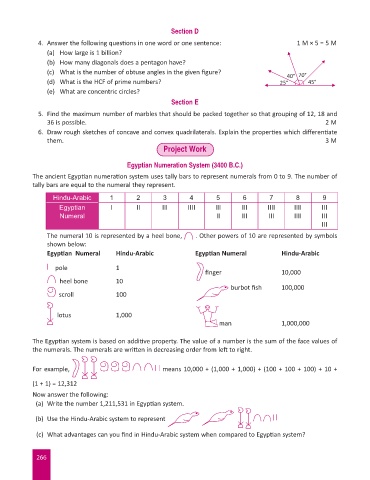
4. Answer the following questions in one word or one sentence: 1 M × 5 = 5 M
(a) How large is 1 billion?
(b) How many diagonals does a pentagon have?
(c) What is the number of obtuse angles in the given figure? 40° 70°
(d) What is the HCF of prime numbers? 25° 45°
(e) What are concentric circles?
Section E
5. Find the maximum number of marbles that should be packed together so that grouping of 12, 18 and
36 is possible. 2 M
6. Draw rough sketches of concave and convex quadrilaterals. Explain the properties which differentiate
them. 3 M
Project Work

Egyptian Numeration System (3400 B.C.)
The ancient Egyptian numeration system uses tally bars to represent numerals from 0 to 9. The number of
tally bars are equal to the numeral they represent.

Hindu-Arabic 1 2 3 4 5 6 7 8 9
Egyptian I II III IIII III III IIII IIII III
Numeral II III III IIII III
III

The numeral 10 is represented by a heel bone, . Other powers of 10 are represented by symbols
shown below:
Egyptian Numeral Hindu-Arabic Egyptian Numeral Hindu-Arabic

pole 1
finger 10,000
heel bone 10
burbot fish 100,000
scroll 100

lotus 1,000
man 1,000,000

The Egyptian system is based on additive property. The value of a number is the sum of the face values of
the numerals. The numerals are written in decreasing order from left to right.

For example, means 10,000 + (1,000 + 1,000) + (100 + 100 + 100) + 10 +

(1 + 1) = 12,312
Now answer the following:
(a) Write the number 1,211,531 in Egyptian system.

(b) Use the Hindu-Arabic system to represent

(c) What advantages can you find in Hindu-Arabic system when compared to Egyptian system?

266