Page 247 - Start Up Mathematics_6

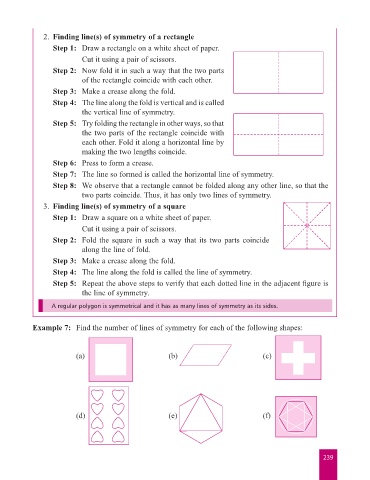
2. Finding line(s) of symmetry of a rectangle

Step 1: Draw a rectangle on a white sheet of paper.
Cut it using a pair of scissors.

Step 2: Now fold it in such a way that the two parts
of the rectangle coincide with each other.

Step 3: Make a crease along the fold.
Step 4: The line along the fold is vertical and is called
the vertical line of symmetry.
Step 5: Try folding the rectangle in other ways, so that
the two parts of the rectangle coincide with
each other. Fold it along a horizontal line by
making the two lengths coincide.
Step 6: Press to form a crease.

Step 7: The line so formed is called the horizontal line of symmetry.
Step 8: We observe that a rectangle cannot be folded along any other line, so that the
two parts coincide. Thus, it has only two lines of symmetry.
3. Finding line(s) of symmetry of a square

Step 1: Draw a square on a white sheet of paper.
Cut it using a pair of scissors.
Step 2: Fold the square in such a way that its two parts coincide
along the line of fold.

Step 3: Make a crease along the fold.
Step 4: The line along the fold is called the line of symmetry.
Step 5: Repeat the above steps to verify that each dotted line in the adjacent figure is
the line of symmetry.

A regular polygon is symmetrical and it has as many lines of symmetry as its sides.

Example 7: Find the number of lines of symmetry for each of the following shapes:

(a) (b) (c)

(d) (e) (f)

239