Page 220 - Start Up Mathematics_6

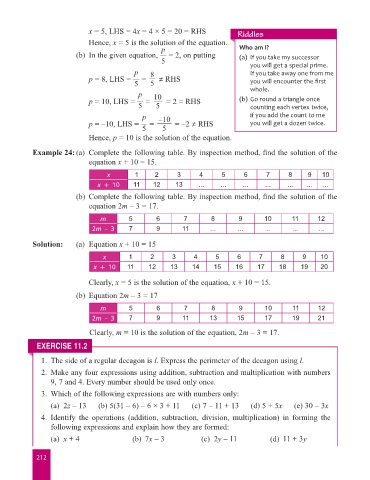
x = 5, LHS = 4x = 4 × 5 = 20 = RHS Riddles

Hence, x = 5 is the solution of the equation.
p Who am I?
(b) In the given equation, = 2, on putting (a) If you take my successor
5 you will get a special prime.
p 8 If you take away one from me
p = 8, LHS = = ≠ RHS
5 5 you will encounter the first
p 10 whole.
p = 10, LHS = = 5 = 2 = RHS (b) Go round a triangle once
5
counting each vertex twice,
p – 10 if you add the count to me
p = –10, LHS = = = –2 ≠ RHS you will get a dozen twice.
5 5
Hence, p = 10 is the solution of the equation.

Example 24: (a) Complete the following table. By inspection method, find the solution of the
equation x + 10 = 15.

x 1 2 3 4 5 6 7 8 9 10
x + 10 11 12 13 ... ... ... ... ... ... ...
(b) Complete the following table. By inspection method, find the solution of the
equation 2m – 3 = 17.

m 5 6 7 8 9 10 11 12
2m – 3 7 9 11 ... ... ... ... ...

Solution: (a) Equation x + 10 = 15

x 1 2 3 4 5 6 7 8 9 10
x + 10 11 12 13 14 15 16 17 18 19 20

Clearly, x = 5 is the solution of the equation, x + 10 = 15.

(b) Equation 2m – 3 = 17

m 5 6 7 8 9 10 11 12
2m – 3 7 9 11 13 15 17 19 21

Clearly, m = 10 is the solution of the equation, 2m – 3 = 17.
EXERCISE 11.2

1. The side of a regular decagon is l. Express the perimeter of the decagon using l.

2. Make any four expressions using addition, subtraction and multiplication with numbers
9, 7 and 4. Every number should be used only once.
3. Which of the following expressions are with numbers only:
(a) 2z – 13 (b) 5(31 – 6) – 6 × 3 + 11 (c) 7 – 11 + 13 (d) 5 + 5x (e) 30 – 3x

4. Identify the operations (addition, subtraction, division, multiplication) in forming the
following expressions and explain how they are formed:
(a) x + 4 (b) 7x – 3 (c) 2y – 11 (d) 11 + 3y

212