Page 22 - Start Up Mathematics_6

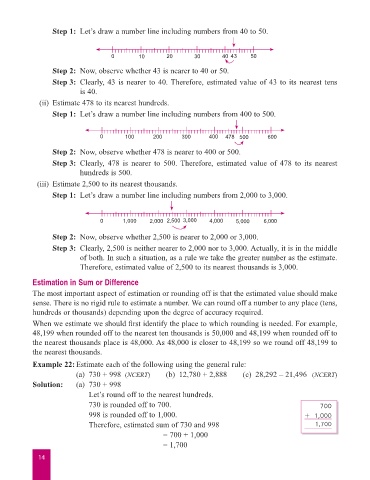
Step 1: Let’s draw a number line including numbers from 40 to 50.

0 10 20 30 40 43 50

Step 2: Now, observe whether 43 is nearer to 40 or 50.
Step 3: Clearly, 43 is nearer to 40. Therefore, estimated value of 43 to its nearest tens
is 40.
(ii) Estimate 478 to its nearest hundreds.
Step 1: Let’s draw a number line including numbers from 400 to 500.

0 100 200 300 400 478 500 600

Step 2: Now, observe whether 478 is nearer to 400 or 500.
Step 3: Clearly, 478 is nearer to 500. Therefore, estimated value of 478 to its nearest
hundreds is 500.

(iii) Estimate 2,500 to its nearest thousands.
Step 1: Let’s draw a number line including numbers from 2,000 to 3,000.

0 1,000 2,000 2,500 3,000 4,000 5,000 6,000

Step 2: Now, observe whether 2,500 is nearer to 2,000 or 3,000.
Step 3: Clearly, 2,500 is neither nearer to 2,000 nor to 3,000. Actually, it is in the middle
of both. In such a situation, as a rule we take the greater number as the estimate.
Therefore, estimated value of 2,500 to its nearest thousands is 3,000.

Estimation in Sum or Difference
The most important aspect of estimation or rounding off is that the estimated value should make
sense. There is no rigid rule to estimate a number. We can round off a number to any place (tens,
hundreds or thousands) depending upon the degree of accuracy required.

When we estimate we should first identify the place to which rounding is needed. For example,
48,199 when rounded off to the nearest ten thousands is 50,000 and 48,199 when rounded off to
the nearest thousands place is 48,000. As 48,000 is closer to 48,199 so we round off 48,199 to
the nearest thousands.
Example 22: Estimate each of the following using the general rule:
(a) 730 + 998 (NCERT) (b) 12,780 + 2,888 (c) 28,292 – 21,496 (NCERT)
Solution: (a) 730 + 998
Let’s round off to the nearest hundreds.
730 is rounded off to 700. 700
998 is rounded off to 1,000. + 1,000
Therefore, estimated sum of 730 and 998 1,700

= 700 + 1,000
= 1,700

14