Page 84 - ICSE Math 4

Let’s write 24 as a product of its prime factors.
Step 1: Start with the smallest prime number that can divide 24 exactly. 24 = 2 × 12

Step 2: Now think of the smallest prime number that can divide 12. 12 = 2 × 6

Put 2 × 6 in place of 12 in the step 1. So, it becomes 24 = 2 × 2 × 6.

We also know that 6 can be divided by 2, i.e., 6 = 2 × 3.

So on pu ng 2 × 3 in place of 6, we have 24 = 2 × 2 × 2 × 3.

So, the prime factors of 24 are 2, 2, 2 and 3, which are all prime numbers.

Prime factors can be obtained by two methods. These methods are factor tree method
and prime factoriza on method.

Let’s learn how to fi nd the prime factors of a given number using these two methods.

Factor Tree Method

In factor tree method, we keep breaking down the given number into factors ll we get
the factors which are all prime numbers. These prime numbers are usually circled. The
shape of a factor tree depends upon how we factorize a number.

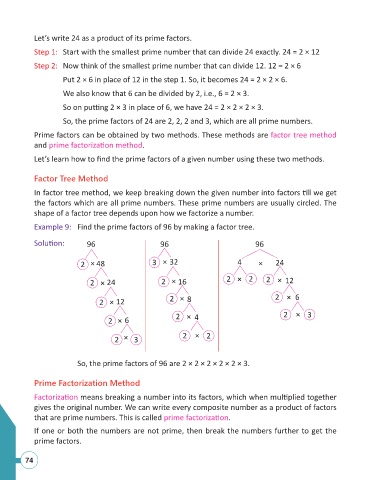
Example 9: Find the prime factors of 96 by making a factor tree.

Solu on: 96 96 96

2 × 48 3 × 32 4 × 24

2 × 24 2 × 16 2 × 2 2 × 12

2 × 12 2 × 8 2 × 6

2 × 4 2 × 3
2 × 6

2 × 3 2 × 2

So, the prime factors of 96 are 2 × 2 × 2 × 2 × 2 × 3.

Prime Factorization Method

Factoriza on means breaking a number into its factors, which when mul plied together
gives the original number. We can write every composite number as a product of factors
that are prime numbers. This is called prime factoriza on.

If one or both the numbers are not prime, then break the numbers further to get the
prime factors.

74